This time of year, a new crop of math majors are stepping off college campuses and into the next phases of their lives. Some go to industry, some into teaching, and some into graduate school. Along any of these paths, one way to continue learning is to watch talks about and using mathematics from the internet, including sources such as TED.com and Vi Hart’s YouTube Chanel. Sadly, many of these talks leave a junior mathematician wanting more. In this post, you will find a sequence of interesting math talks that have been extended by further literature research by graduating seniors for your continued edification. The internet awaits – enjoy!
Table of Contents (Titles are jump-links in the post):
- Mathemagic (Guest Author: Adam Bengfort)
- Human-made Fractals (Guest Author: Alex Odenkirk)
- Modeling Love (Guest Author: Cody Hansen)
- WolframAlpha and the Future of Computing (Guest Author: Dan Shultz)
- Math’s Ability to Make Sense of War (Guest Author: David Devore)
- Visualizing Data (Guest Author: Devon White)
- Thought Experiments that Boil Down to “What If?” (Guest Author: Emily Leazer)
- To Infinity and Beyond (Guest Author: Eric Josupait)
- A Reality Check on Renewables (Guest Author: Eyoel Berhane)
- Applications of Algorithms (Guest Author: Grace Vente)
- The Expansive Possibilities of Teaching Statistics Before Calculus (Guest Author: Jeff Prior)
- Fibonacci Numbers and Teaching Math as Art (Guest Author: Jessica Launius)
- Using Mathematics to Generate Music (Guest Author: Kelly McCurry)
- Why Mathematics Instruction IS Necessary (Guest Author: Kimmy Grimmer)
- From Sonars to Music to Cryptography (Guest Author: Lauren Carlson)
- Classroom Makeover (Guest Author: Mike Hanson)
- Models to Represent Hyperbolic Geometry (Guest Author: Paul Landahl)
- Symmetry in Science (Guest Author: Rebecca Post)
- High School Math Focus: Calculus or Statistics? (Guest Author: Rebecca Sund)
- Powerful Statistics… Powerful Applications (Guest Author: Reed Kottke)
- Teaching with Computers (Guest Author: Williams Payne)
~
Mathemagic
Guest Author: Adam Bengfort
In this TED presentation, given by Arthur Benjamin, titled A Display of “Mathemagic,” the speaker combines his love for numbers and magic. Throughout the show, Benjamin gives amazing examples of his ability to compute rather difficult mathematical solutions without doing pen and paper arithmetic. He begins by simply multiplying together two digit numbers in his head and then moves on to calculating the squares of numbers. He starts with two digit squares and moves on to three, four, and even a five digit numbers before the end of the presentation. Benjamin’s display also includes a segment in which he is told a specific date (year, month, and day) and is able to say what day of the week it was. He completes all of his calculations very quickly and has intrigued me to look into his process.
Due to the presentation being in the form of a magic show, Benjamin does not give much clue into how he is able to complete his calculations so quickly in his head. To the uninformed viewers of the TED presentation, his ability to complete mental arithmetic is sold as magic. However, as part of the mathematical community, we know that he must be using some sort of technique to complete his mental computations. While completing his five digits square, he does some thinking out loud using word associations and of course, mental math. His process is fast and difficult to follow, so I have been inspired to dig further into finding solutions to squares. I feel this to be the most interesting and most applicable part of his show.
Through research, I have learned a simply way to square two digit numbers more easily. The formula is given as A2 = (A − d)(A + d) + d2. Here, d is the numerical distance away from the original number A used in order to break the problem up into easier parts. For example, 132 can be calculated by multiplying ten by sixteen, and then adding three squared to the result. So using d = 3 we have 132 = (13 – 3)(13 + 3) + 32 = (10)(16) + 9 = 160 + 9 = 169. I have found it most helpful to have d equal something that will round the A value to the nearest 10. The equation works both with rounding up and rounding down.
Squaring three digit numbers also follows the same formula as above. For Example, 7062 = (706 – 6)(706 +6) + 62 = (700)(712) + 36 = 498,400 + 36 = 498,436. Now if this isn’t complicated enough, many times a three digit square could involve a d value that is a two digit number. This would then lead to needing to do a two digit square within the three digit problem. For example, 3142 = (314 – 14)(314 + 14) + 142 = (300)(328) + 142. Now the first term can be multiplied and the 142 can be found by two digit squaring leading to the final solution of 98,596. This process is diagramed here.
Although it is debatable whether or not this multiplication method is “easy,” it is clear that with some practice, this will make squaring numbers more straightforward. Numbers of many digits can be broken down using that very first formula of A2 = (A − d)(A + d) + d2. Through practice with two digit and three digit squares, this mathemagical ability is well within sight.
What’s next? My sources listed below include many mathematical techniques that could help us each improve in our day to day computations. I specifically am interested in learning better techniques to develop my mental math skills in the areas of division. I feel this area has been lacking and I often find myself needing to pull out a calculator. These sources also give techniques for estimation, advanced multiplication, addition and subtraction, memorizing numbers, and much more. Continuing with the research described above, I would be interested to see if the same technique for squaring can be used in arithmetic other than base 10. Does a different pattern form that will lead to a new technique? This area of mathematical thought seems to be relatively unexplored. Perhaps with a bit of inquiry, an entirely new field of mathemagic will be born
Sources:
Benjamin, A.T. and Shermer, M. (206). Secrets of Mental Math. New York, NY. Random House, Inc.
Benjamin, A. T. (2012). Squaring, Cubing, and Cube Rooting. College Mathematics Journal, 43(1), 58-63.
Benjamin, A. T. (2005, February). Arthur Benjamin: A performance of “Mathemagic” [Video File]. Retrieved from http://www.ted.com/talks/arthur_benjamin_does_mathemagic?language=en#t-900684
Image Source: Benjamin, A. T. (2005, February). Arthur Benjamin: A performance of “Mathemagic” [Video File]. Retrieved from http://www.ted.com/talks/arthur_benjamin_does_mathemagic?language=en#t-900684
~
Human-made Fractals
Guest Author: Alex Odenkirk
The activity of forcing complex mathematical ideas onto simple scenarios found in nature or society has become a popular one of late, particularly regarding topics like the Golden Ratio and the Fibonacci Sequence. Not all of these comparisons are a stretch, however, as Ron Eglash discovered in the 1980’s while looking at top-down pictures of villages in Africa. He quickly noticed that the villages’ streets and houses were organized according to recursive geometric patterns such as spirals and fractals. After traveling to Africa and visiting villages all over the continent, he began to find that not only were the villages designed so that each section of the village was a smaller version of the larger design of the village, but that art in the homes of the Africans’ also contained many recursive patterns. Eglash discussed these phenomenon at his TED talk in 2007, which can be found here: https://www.ted.com/talks/ron_eglash_on_african_fractals#t-660714.
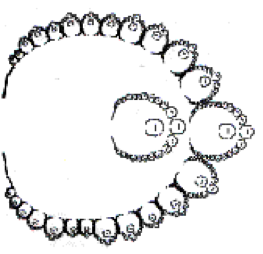
A ring-shaped village composed of smaller rings of houses http://classes.yale.edu/fractals/panorama/Architecture/AfricanArch/BaIla.html
This discovery prompts the questions of where else fractals might be found, either in human constructions or in nature, and what purposes might they serve. Eglash mentions several such places in his talk, like certain African fences woven out of straw using fractal patterns that are tighter than normal patterns, allowing less dirt and wind to come through the fence. In a separate article, Eglash describes the practical applications of fractals in a building designed by TED-Fellow Xavier Vilalta, saying, “The Lideta Mercato design is beautiful, of course, but for me it is especially satisfying to see how [Xavier] has managed to nurture the fractal tradition from Africa into this new form, and help the next generation carry that into the future… It’s more than just aesthetics: the scaling structure provides a practical means of providing airflow and improving the building’s ecological fit.”
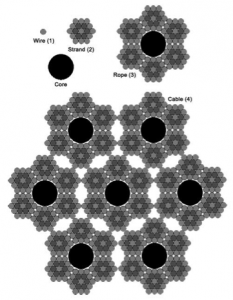
Others have begun to take note of the presence of fractals in the world, and perhaps none more so than the group of math professors and professionals referring to themselves as the “Fractal Foundation.” The Fractal Foundation is dedicated to studying and teaching the general populace about fractals. An entire page of their website is dedicated to the description of how fractals are used in the construction of modern suspension-bridges, as each cable on the bridge is comprised of a series of smaller woven cables, which are in turn comprised of even smaller cables, etc. The whole thing, when viewed by a horizontal cross section, looks like a simple fractal.
There are even stranger, yet equally useful, fractals to be found outside the realm of architecture. Fractal geometry has been found to be incredibly useful in measuring distances accurately when the object being measured is very convoluted. In their article “Use Of Fractal Theory in Neuroscience”, Eduardo Fernandez and Herbert Jelinek discuss the advantages and disadvantages of using fractal dimensions to measure the lengths of nerves. Nerves are very small and wind about in odd directions, and thus are near impossible to measure by conventional means. Their length can, however, be accurately estimated using certain fractals and regression models. This has led to advances in neuroscience, as biologists can better calculate the speed at which nerve impulses are actually traveling through a nerve.
In addition to being generally useful, many fractals can also be said to be aesthetically pleasing. This claim can be substantiated by the astonishing number of fractals to be found in the art of cultures all over the world. In Indian cultures, temples often have towers comprised of smaller, tower-shaped structures. These towers, according to William Jackson, the author of Heaven’s Fractal Net: Retrieving Lost Visions in the Humanities, represent, “the infinite rising levels of existence and consciousness, expanding sizes rising toward transcendence above.” Recursive, self-similar geometric patterns can also be found on the internal and external artwork and design of the Eiffel Tower, St. Peter’s Basilica, and other famous European structures.
It can be concluded, based on the work of Ron Eglash and other researchers of fractals, that the use of complex geometric patterns is a universal human idea, and that it may have roots in more than just the creation of aesthetically pleasing buildings and art. There is no doubt more applications of fractal geometry exist in the world, just waiting for enterprising mathematicians to stop by and view things in a slightly different way than most.
Sources:
Architecture infused with fractals: How TED speaker Ron Eglash inspired architect Xavier Vilalta. (2013, October 25). Retrieved April 28, 2015, from http://blog.ted.com/architecture-infused-with-fractals-ron-eglash-and-xavier-vilalta/
Eglash, R. (2007, June 1). The fractals at the heart of African designs. Retrieved April 28, 2015, from https://www.ted.com/talks/ron_eglash_on_african_fractals#t-660714
Fernandez, E., & Jelinek, H. (2001, January 1). Use of Fractal Theory in Neuroscience: Methods, Advantages, and Potential Problems. Retrieved April 28, 2015, from http://webcache.googleusercontent.com/search?q=cache:7ANpsK0DQ0gJ:www.researchgate.net/profile/Herbert_Jelinek2/publication/11876325_Use_of_fractal_theory_in_neuroscience_methods_advantages_and_potential_problems/links/00b7d52b71e433d7f7000000.pdf &cd=1&hl=en&ct=clnk&gl=us
Fractal Applications. (n.d.). Retrieved April 28, 2015, from http://fractalfoundation.org/OFC/OFC-12-1.html
Fractals in Architecture. (n.d.). Retrieved April 28, 2015, from http://classes.yale.edu/fractals/panorama/Architecture/Arch/Arch.html
~
Modeling Love
Guest Author: Cody Hansen
Hannah Fry’s TED talk titled Mathematics of Love is an interesting talk about how we can use mathematics to guide our romantic life. There are 3 components to this talk, with the first devoted to maximizing your chance of successfully finding a romantic partner through online dating. The second part is an application of optimal stopping theory, where the goal is to have the highest chance of finding the best romantic partner. The third portion of the talk is about modeling a couple’s conversation over turns of conversation amongst the couple.
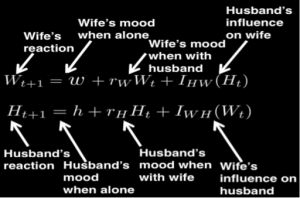
In this third section of her talk, Hannah Fry discusses the work of John Gottman and James Murray on this subject. According to Fry, the love difference equations look like:
Fry describes the influence function as a step function with a positivity threshold labeled T+ and a negativity threshold T-. At these thresholds, large jumps in either positivity or negativity occur that will drastically affect the path of the conversation.
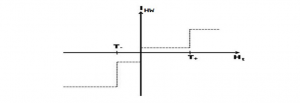
Modified image taken from http://www.brainpickings.org/2015/02/18/hannah-fry-the-mathematics-of-love/
More recent research by Gottman and Murray suggests this model may be incorrect. They found the bilinear model fits their data better than the step function and it has fewer parameters.
Gottman also prefers to use a system of differential equations rather than difference equations to model the flow of conversation over time instead of turns.
 Now, I’m going to rewrite Gottman and Murray’s equations to look more like Fry’s, but I will modify the influence equation into a heaviside equation that behaves like the bilinear model.
Now, I’m going to rewrite Gottman and Murray’s equations to look more like Fry’s, but I will modify the influence equation into a heaviside equation that behaves like the bilinear model.![]() The unknown constants a and b can be thought of as the emotional effect the husband has on the wife. The unknown constants c and d can be thought of as the emotional effect the wife has on the husband.
The unknown constants a and b can be thought of as the emotional effect the husband has on the wife. The unknown constants c and d can be thought of as the emotional effect the wife has on the husband.
When H(t) is positive, a is the coefficient and when H(t) is negative, b is the coefficient.
When W(t) is positive, c is the coefficient and when W(t) is negative, d is the coefficient.
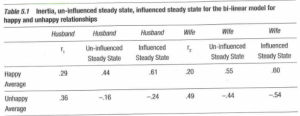
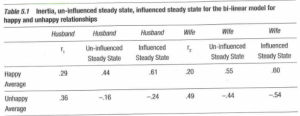
The following is a table of values for some of these coefficients that that Gottman and Murray have found in their studies.
In order we are given r_H, which is the husbands reaction coefficient to his own emotional displacement from his normal steady state, H(0) which is his normal emotional equilibrium that is uninfluenced by his wife, an equilibrium point H(t) where H'(t) = 0 thus meaning no more emotional change after being influenced by his wife. The wife’s corresponding information is given next. We are not giving any of the slope coefficients a,b,c,d. These values are averages and thus the unknown constants coefficients a,b,c,d should each be thought of an average value of emotional effect.
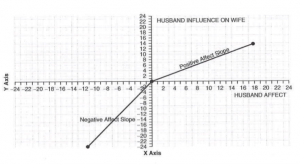
One thing is also important to note, Gottman and Murray studied “Happy Couples” and “Unhappy Couples” meaning that we only have information about the couples who primarily exist in the top right quadrant and those who primarily exist in the bottom left.
This means our model is limited to the first and third quadrants. This limitation simplifies our model equations into two different systems of equations, neither set of systems containing the heaviside as a and c are turned on in quadrant one and b and d are turned on in quadrant 3.
 With this information we can solve for a,c of the average happy couple and the b,d of the unhappy couple.
With this information we can solve for a,c of the average happy couple and the b,d of the unhappy couple.
Let’s find the a,c of the average happy couple.
We know that our happy couple reaches an equilibrium, so at some t, W'(t) = 0 and H'(t)=0. At this future t, W(t) = 0.60 and H(t) = 0.61. If we also assume that our average husband and average wife come into this conversation at their un-influenced steady state then we know W(0) = 0.55 and H(0) = 0.44. We also have an r_W = 0.20 and r_H = 0.29, these values are the emotional response due to being away from one’s own un-influenced emotional steady state. Plugging all these values in we have
Solving them we get a = 0.0164 and c = 0.0822. If we solve for b and d using the corresponding average unhappy couple information we find that b = 0.2042 and d = 0.0533.
In the case of the average happy couple, the husband’s emotional state has a lower effect on the wife’s emotional state than the wife’s has on the husband’s. In the average unhappy couple, this situation is reversed and the husband’s emotional state has a larger effect on the wife’s emotional state.
In the end, Hannah Fry is correct. Mathematics can tell us something about love. In a happy couple both partners will have a positive disposition by themselves and interactions with their partners will improve their dispositions. Within these relationships the wife’s emotional disposition has a larger effect on her husband than vice versa. If you men want to be in a happy relationship, keep your wife happy as it will make you happier.
In an unhappy relationship this relationship is reversed. Both partners have a negative emotional steady state, their dispositions are worsened by interactions with one another and the husband’s mood has the larger effect on the wife’s.
Mathematics has provided some evidence for things we believe true about successful and unsuccessful couples. In healthy relationship the participants moods are improved with the interaction of each other but depend first and foremost on acquiring one’s own happiness first. In unhealthy relationships things go from bad to worse and don’t get better. There it is, (some) of the mathematics behind love.
Sources:
Fry, Hannah. “The mathematics of love”. TEDxBinghamtonUniversity. April 2014. Lecture.
Popova, Maria. “What Mathematics Reveals About the Secret of Lasting Relationships and the Myth of Compromise.” Brain Pickings. n.d. Web. 10 Apr. 2015.
Gottman, John Mordechai. Principia Amoris: The New Science of Love. New York: Routledge, 2015. Print.
~
WolframAlpha and the Future of Computing
Guest Author: Dan Shultz
In his 2010 TED talk titled Computing a theory of all knowledge, Stephen Wolfram introduced us to his mission of better understanding the computational universe. He showed us tools he had created such as Mathematica, Wolfram Alpha, and A New Kind of Science to help solve some of the biggest questions we have about nature today.
Using a simple program with elementary rules, Wolfram shows how a simple system can create an incredibly complex system that is fundamentally irreducible; just like structures we see in nature.
He calls this his Principle of Computational Equivalence – even incredibly simple systems can do incredibly complex computations. He believes this has a real importance to the science world as it doesn’t take much much technology or biological evolution to do arbitrary computations. This claims that this tells us that simple rules can tell us as about things as complex as evolution, economy, intelligence and even the universe.
Well you may be wondering, how does this tell us anything in a less abstract way? Wolfram goes on to present his latest app Wolfram Alpha. Eventually beginning as a project to emulate the brain, he ended up with an enormous computational system that can turn everyday language into meaningful computations, patterns, and things we may not have thought to look at.
Not only has this type of computational engine helped every math major throughout college, it demonstrates a computer’s ability to use data to come to conclusions that were not directly programmed in. This is where Wolfram sees the beauty of WolframAlpha’s ability to use the simple, yet irreducible patterns. Instead of relying on the knowledge base of our civilization, it is able to go out into the computational universe and discover on the fly. This is where it differs itself from other supercomputers, such as IBM’s Watson that played, and won in Jeopardy!. In comparison, Watson was built to answer very specific types of questions and was even criticized that if you asked it was time it was, “it won’t have an answer” (Baker, 152). Not that Watson isn’t an impressive feat of its own, but it is a great example of how WolframAlpha excels in using answering questions it was not explicitly programmed to answer.
What does WolframAlpha have in store for the future? Well it is certainly unknown at this time, but it will be exciting to see and discover more of these simple, yet irreducible patterns, some of which may relate closely to the universe we live in.
Sources:
Baker, Stephen. Final Jeopardy: Man Vs. Machine And The Quest To Know Everything.
Boston : Houghton Mifflin Harcourt, 2011.
Kelly, John. Smart Machines : IBM’s Watson And The Era Of Cognitive Computing.
Wolfram, Stephen. A New Kind Of Science. Champaign, IL : Wolfram Media, ©2002.
~
Math’s Ability to Make Sense of War
Guest Author: David Devore
Sean Gourley and his team tackled the question can today’s modern conflict be explained. During the time of Sean’s investigation the Iraq conflict was in full swing and Sean and his team noticed every day accounts of different attacks and how many causalities. The Iraq conflict was one of many “modern conflicts” they investigate. Gourely’s team defined modern conflicts as insurgencies that happen around the globe (Patterson). This was the basis of their investigation, trying to find a mathematical pattern between the frequency of attacks and the amount of causalities. After Sean and his team started to crunch the raw data they came to a startling conclusion. Even with the chaos of war and the large amount of data they produced a precise mathematical distribution that all data points fell upon (Patterson). Their team was stumped by how there was order in the conflict in Iraq but when compared to other conflicts in countries like Afghanistan, Colombia, Senegal, Sierra Leone, etc. and every conflict had the same underlying structure creating order in all of these conflicts. All of these conflicts followed the same distribution pattern which are all power law distributions (Meier). But why stop there? Can a power law distribution help create mathematical patterns in different conflicts? Maybe it can be used to model other real world events?
When asking this question one has to begin looking at the source of these patterns, the power-law distribution. The basic definition of the power law is that it is a function with a relationship between two quantities where one of the quantities is the power of another. For Sean’s study the two quantities where the number killed raised to the power of the “organizational structure” or the frequency of attacks (Patterson). With the understanding of the power-law the next step was to explore different areas of war that the power-law has been able to model. Little did I know that this phenomenon with power-law distribution was discovered back in 1948 by Lewis F. Richardson, an English meteorologist and physicist. Richardson found that the severity of interstate wars can be modeled with a power-law distribution (Meier). A professor at Harvard University wanted to see if his findings still hold up from today’s conflicts and attempted to make a distribution of severity of interstate wars from 1820 to 1997 (Cederman 135-136). His name was Lars-Erik Cederman and he published his work in “Modeling the Size of Wars: From Billiards to Sandpile”.
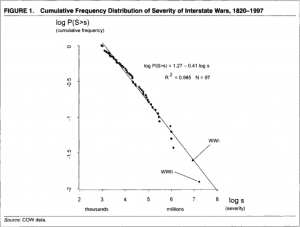
Image From: Lars-Erik Cederman’s paper “Modeling the Size of Wars: From Billiard Balls to Sandpiles” pg 136
Another application of the power-law distribution was used by Oscar Becerra, Neil Johnson, Patrick Meier, Jorge Restrepo and Michael Spagat to create a distribution for natural disasters. This team focused on the magnitude, amount killed, and the frequency of different natural disasters throughout the world. They were able to create a power law distribution, which fit with past results when looking at wars and terrorist events, for different continents and regions with those continents. After looking at these studies we can see that the power-law distribution is a powerful tool that can help us better understand different real world events and phenomenons.
Sources:
Cederman, Lars-Erik. “Modeling the Size of Wars: From Billiard Balls to Sandpiles.” The American Political Science Review 97.1 (2003): 135-50. JSTOR. Web. 27 Apr. 2015.
Meier, Patrick. “The Mathematics of War: On Earthquakes and Conflicts.”IRevolutions. N.p., 21 Oct. 2011. Web. 27 Apr. 2015.
Patterson, Lindsay. “Sean Gourley: The Mathematics of War | EarthSky.org.” EarthSky. Human World, 25 Oct. 2010. Web. 27 Apr. 2015.
~
Visualizing Data
Guest Author: Devon White
In his Ted Talk The Beauty of Data Visualization, David McCandless talks about how a simple re-representation of data can really change how we feel about a situation. When humans look at a data set, it is easy to let the numbers not sink and move on, without letting the data move us. McCandless says that when we represent this data in a striking way, the numbers of behind the data can start to make humans change their perspective and really think about what really matters.
 Recently talked about in the media and in technological circles around the world, the term “big data” has been coined as a way of talking about the vast amount of information that can be gathered about any user. If you’re on the internet, there is someone gathering your information. If you sign up for a rewards program or give away your email address at a store, there is someone gathering your data. There’s no telling what those companies are doing with your data. When the data is gathered, it doesn’t look much cleaner than a spreadsheet filled to the brim with numbers. If data scientists can take McCandless’ advice on the representation of data, they can really influence how America’s corporations.
Recently talked about in the media and in technological circles around the world, the term “big data” has been coined as a way of talking about the vast amount of information that can be gathered about any user. If you’re on the internet, there is someone gathering your information. If you sign up for a rewards program or give away your email address at a store, there is someone gathering your data. There’s no telling what those companies are doing with your data. When the data is gathered, it doesn’t look much cleaner than a spreadsheet filled to the brim with numbers. If data scientists can take McCandless’ advice on the representation of data, they can really influence how America’s corporations.
McCandless’ entire talk is centered on the visualization of data that is relatively useless to our society. However if we take some of the ideas that he talks about, and apply them to data that is more than just merely interesting, we may find that the art of data visualization may be able to change our world.
Sources:
“Data Mining.” Encyclopedia of Computer Science. Eds. Edwin D. Reilly et al. Hoboken: Wiley, 2003. Credo Reference. Web. 28 Apr 2015.
“Google’s Infrastructure.” Wired.com. N.p., n.d. Web. 27 Apr. 2015.
Hogan, Mél, and Tamara Shepherd. “Information Ownership and Materiality in an Age of Big Data Surveillance.” JSTOR. Pennsylvania State University Press, n.d. Web. 27 Apr. 2015.
~
Thought Experiments that Boil Down to “What If?”
Guest Author: Emily Leazer
 Thought experiments can be a powerful tool, and it seems as though these are Randall Munroe’s specialty. Throughout Munroe’s TED talk he discusses how on his website there is a place for people to ask hypothetical questions for him that he will try to answer using math, science, and comics. First, he introduces a question he received asking what would happen if a baseball were to be pitched at 90% the speed of light. From there he describes what would happen mathematically and scientifically, explaining how it ultimately would result in huge cloud over the entire area. Then he moves onto another question asking how big Google’s data warehouse would have to be if all the data was stored on punch cards. He continues to think out loud and explains how in order to answer this, he looked into money spent, power and electricity used, and square footage of data centers, along with pretty much anything he could get his hands on in order to provide his “educated guess.”
Thought experiments can be a powerful tool, and it seems as though these are Randall Munroe’s specialty. Throughout Munroe’s TED talk he discusses how on his website there is a place for people to ask hypothetical questions for him that he will try to answer using math, science, and comics. First, he introduces a question he received asking what would happen if a baseball were to be pitched at 90% the speed of light. From there he describes what would happen mathematically and scientifically, explaining how it ultimately would result in huge cloud over the entire area. Then he moves onto another question asking how big Google’s data warehouse would have to be if all the data was stored on punch cards. He continues to think out loud and explains how in order to answer this, he looked into money spent, power and electricity used, and square footage of data centers, along with pretty much anything he could get his hands on in order to provide his “educated guess.”
Although the answers he comes up with are interesting, the most intriguing part is his thought process. As he states in the video, he is able to put together things he knows in order find out things he doesn’t know. The math behind solving it can be pretty simple, but the most important part is how to attack it. Where can you look? Who can you ask? What data is already out there? This made me curious what other people have done with their own thought experiments. Has anyone else tried this type of procedure?
After researching carefully, I found many mathematicians and scientists have indeed looked into solving problems in this manner. Specifically, I looked into Schrodinger’s cat thought experiment that was developed in 1935. This involves using a cat and a radioactive atom inside of an enclosed box in order to find out whether the atom will decay or release poisonous gas. Until the result is shown, the state of the cat is superposed. Regardless of the results, the process of the thought experiment is where the connection to Munroe comes in. Both Schrodinger and Munroe have to continually ask themselves the question, “what if?”  In Munroe’s case, he in inquiring about what his further estimates regarding Google should be, but in Schrodinger’s experiment he is looking to find out what part of the set-up went wrong and which part went right. From there he can look into fixing the structure of his research. Without asking “what if?” in both situations, the ability to explore options and make different connections becomes difficult.
In Munroe’s case, he in inquiring about what his further estimates regarding Google should be, but in Schrodinger’s experiment he is looking to find out what part of the set-up went wrong and which part went right. From there he can look into fixing the structure of his research. Without asking “what if?” in both situations, the ability to explore options and make different connections becomes difficult.
Schrodinger’s experiment further extends to connecting it further to playing cards. According to physics, we should be able to stand up a card on its side and never worrying about it falling. But, as we know it could fall both left and right. Until we know what side it has fallen to, it is also in a superposed state. The connections made throughout answering questions can be very substantial and always lead you to wanting to know more; wanting to know “what if?”
Sources:
Munroe, Randall. “Comics That Ask, “what If?”” TED.com. 1 Mar. 2014. Web. <https://www.ted.com/talks/randall_munroe_comics_that_ask_what_if?language=en>.
Tarlaci, Sultan. “The Quantum Measurement Problem: How To Rescue A Cat From Schizophrenia?.”Neuroquantology 10.3 (2012): 519-531. Academic Search Complete. Web. 1 Apr. 2015.
WOLFSON, ELIJAH. “Everything You Never Knew You Wanted To Know.” Newsweek Global 163.14 (2014): 58-59. Academic Search Complete. Web. 31 Mar. 2015.
~
To Infinity and Beyond
Guest Author: Eric Josupait
Quick, what is the biggest number you can think of? One million? Nine hundred trillion? Infinity? Depending on who you ask you will get different responses and I would venture to say that the answer of infinity would come up quite a bit. I know that’s how I would have answered this question back in middle school and hig h school. That is, until I learned that infinity is not a number, but rather a concept or idea. Soon after this discovery I learned that there are different sizes of infinity and that there are infinite sizes of infinity. In Dennis Wildefogel’s video, “ How big is infinity?”, which is linked here http://ed.ted.com/lessons/how-big-is-infinity, he provides a brief synopsis of this idea. I will be working to extend this idea and better understand the work of Georg Cantor.
h school. That is, until I learned that infinity is not a number, but rather a concept or idea. Soon after this discovery I learned that there are different sizes of infinity and that there are infinite sizes of infinity. In Dennis Wildefogel’s video, “ How big is infinity?”, which is linked here http://ed.ted.com/lessons/how-big-is-infinity, he provides a brief synopsis of this idea. I will be working to extend this idea and better understand the work of Georg Cantor.
When we talk about different sizes of infinity the person who’s work that needs to be mentioned is Georg Cantor. Cantor is the one who is credited with developing the mathematics of infinity and particularly the phenomenon that there are different sizes of infinity. Generally, if we want two sets to have the same size then there must be a one-to-one correspondence between the two sets. Cantor managed to show that the set of Real numbers do not have a one-to-one correspondence with the set of natural numbers. These are both infinite sets, but not having a one-to-one correspondence shows the different sizes between the sets. Cantor proved this using what is known now as the diagonalization argument, which comes from the idea that a new rational number can always be created. When creating a real number we can always make sure that the nth digit in our new number is different from the nth digit in the nth row. This makes sure that there is always a new number within the set of real numbers. The set of real numbers is an infinitely countable, but Cantor’s diagonalization argument proves that there are infinite different sizes of infinity!
Cantor also uses the Hebrew symbol aleph, ![]() , and what he calls aleph naught,
, and what he calls aleph naught, ![]() , to
, to  indicate the cardinality of the natural numbers. In mathematics when we talk about aleph we are talking about the size of sets. Aleph naught is what we use to indicate the largest countable infinite set. However, he also showed that there are numbers bigger than aleph naught and these numbers were said to be uncountable. This would include the set of real numbers and this ties back to the diagonalization argument. This is also associated with the Cantor’s continuum hypothesis, which shows that the set of integers is smaller than the set of real numbers. To put in another way, the aleph naught of the integers will always be smaller than any possible cardinality of the real numbers.
indicate the cardinality of the natural numbers. In mathematics when we talk about aleph we are talking about the size of sets. Aleph naught is what we use to indicate the largest countable infinite set. However, he also showed that there are numbers bigger than aleph naught and these numbers were said to be uncountable. This would include the set of real numbers and this ties back to the diagonalization argument. This is also associated with the Cantor’s continuum hypothesis, which shows that the set of integers is smaller than the set of real numbers. To put in another way, the aleph naught of the integers will always be smaller than any possible cardinality of the real numbers.
Starting with infinity and then examining the idea that there are infinite sizes of infinity seems counterintuitive. Georg Cantor lead the way in mathematics for understanding what infinity is and furthermore that there are infinite sizes of infinity. Cantor’s continuum hypothesis and diagonalization argument has helped provide a foundation for modern mathematics and particularly calculus since calculus is rooted around the idea of infinity. Understanding infinity has allowed mathematics to move into a new realm and we have Georg Cantor to thank for that.
Sources:
Asperό, David, Paul Larson, and Justin Moore. “Forcing Axioms And The Continuum Hypothesis.” Acta Mathematica 210.1 (2013): 1-29. Academic Search Complete. Web. 29 Apr. 2015.
Gray, Robert. “Georg Cantor and Transcendental Numbers” The American Mathematical Monthly. Mathematical Association of America. Nov. 1994. 819-832. Web. 31 Mar. 2015.
Kolar, Vida Manfreda, Cadez, Tatjana Hodnik “ Analysis of Factors Influencing the Understanding of the Concept of Infinity” Educational Studies in Mathematics, 2001. Springer. 389-412. Web. 31 Mar. 2015.
N.d. Mathmunch.org. Web. 30 Apr. 2015.
N.d. Wikipedia.org. Web. 30 Apr. 2015.
Nielsen, Mark J. “That Which Surpasses All Understanding”: The Limitations Of Human Thought.” Dialogue: A Journal Of Mormon Thought 42.3 (2009): 1-20. Academic Search Complete. Web. 10 Apr. 2015.
Paoletti, Teo J. “Are All Infinities Created Equal?” The Mathematics Teacher. The National Counsel of Teachers of Mathematics, Sept. 2013. 98-103. Web. 31 Mar. 2015.
Tall, David. “Natural and Formal Infinities”. Educational Studies in Mathematics, 2001. Springer. 199-238. Web. 31 Mar. 2015.
~
A Reality Check on Renewables
Guest Author: Eyoel Berhane
Dr. Mackay’s presentation on the “A reality check on renewables” brought me back to my Fall Term class of 2014, where I had a class discussing similarly jarring scales that renewable energy needed to be to meet world consumption. I wanted to follow up on some of Mackay’s points on the truth behind alternative forms of energy use. Scoping various sources, I managed to find several that inquired on the reality of a “clean energy market”. He had an excellent point on the impractical area use for biofuel production: one source attempts to evaluate the sensibleness of the U.S. corn ethanol market. It finds that with its already high water and labor demand compared to regular crops, the fact it is often infused with gasoline anyways, and the area needed for the high-cost production (without subsidies), ethanol becomes a difficult marketable energy alternative (Somma, Lobkowicz and Deason, 2009). The study uses static numbers to approximate the productivity of renewables compared to gas,
Mackay discusses how the effectiveness of renewable energy production compared to fossil fuels comes in overcoming the challenges. He displays a graph of countries and their energy consumption per person related to people/km2. He finds that most energy changes over the last 15 years for some countries travel up-right of the graph (meaning more energy and more people per area). He then presents a scale of model of alternative transportation vehicles compared to the normal UK energy consumption, scaling by a form of lifestyle change and efficiency. He hopes to distinguish a realistic representation of alternative travel compared to options he felt most people would find unsatisfying. For example, he supposes that electric vehicles were the more realistic choices compared to other forms of travel, being in the center of efficacy vs. lifestyle change. Most of Mackay’s research depends on static stable numbers, while stressing that energy consumption and the demand has been growing larger.
That led me to rummage through one research article capturing Brazil’s efforts in progressing to a greener energy market. With the addition of share in the use and production of wind and solar energy, personal interviews were gathered to reflect public opinion of renewables. Quantifying the microclimates and surface topography changes in the large country’s area zones (Northeast, Southwest, Midwest, etc) has proven difficult to assess the optimization of potential energy, especially for hydropower (Martins & Pereira, 2011). That underlines the biggest concern when it came to a renewable market: encouraging incentive. As financial and energy institutions recognize the instability and intense management of renewable energy markets, it’s of little surprise such renewables are restricted to urban and experimental settings (Martins & Pereira, 2011).
Countries like Mexico and Finland has been very active in the renewable energy market with its hydropower and solar energy markets. Finland is seeing large amounts of hydropower and wind power powering sections of its energy market, with 90% hydropower generated from large-scale facilities and wind energy set to increase by 6TWh by 2020 (Aslani, Helo and Naaranoja 2013). Mexico is one of the top 5 countries that have the best solar-power generation prospects in the world, at least for photovoltaic (PV) technology. This figure below illustrates certain forms of mathematical modeling of renewable energy projection, estimating the solar irradiation Mexico is exposed to daily (Alemán-Nava et al. 2014).
 What is important to take away from such research is that having the ability to approximate quantities of energy use across wide areas over time, as scientists have been doing for decades, helps researchers compare the current global energy market with those for projected clean energy markets. If the numbers are able to speak favorably of certain conditions and prospects for clean energy, data projections assist in narrowing down future plans that fit ideal standards for renewables. The ability to understand the optimization of wind energy across the globe, or solar irradiation spread, through charts and quantity maps illustrate real-life models of world challenges for engineers and policy-makers.
What is important to take away from such research is that having the ability to approximate quantities of energy use across wide areas over time, as scientists have been doing for decades, helps researchers compare the current global energy market with those for projected clean energy markets. If the numbers are able to speak favorably of certain conditions and prospects for clean energy, data projections assist in narrowing down future plans that fit ideal standards for renewables. The ability to understand the optimization of wind energy across the globe, or solar irradiation spread, through charts and quantity maps illustrate real-life models of world challenges for engineers and policy-makers.
Sources:
Alemán-Nava GS, Casiano-Flores VH, Cárdenas-Chávez DL, Díaz-Chavez R, Scarlat N, Mahlknecht J, Dallemand J, Parra R. 2014. Renewable energy research progress in Mexico: A review. Renewable and Sustainable Energy Reviews 32(0):140-53.
Aslani A, Helo P, Naaranoja M. 2013. Evaluation of renewable energy development in power generation in Finland. Journal of Renewable & Sustainable Energy 5(6):063132.
Jacobson MZ and Archer CL. 2012. Saturation wind power potential and its implications for wind energy. Proc Natl Acad Sci U S A 109(39):15679-84.
Somma D, Lobkowicz H, Deason JP. 2010. Growing America’s fuel: An analysis of corn and cellulosic ethanol feasibility in the united states. Clean Technologies & Environmental Policy 12(4):373-80.
~
Applications of Algorithms
Guest Author: Grace Vente
In Kevin Slavin’s talk, “How Algorithms Shape the World ”, Slavin explains that math is becoming the “physics of our culture” via algorithms. Websites such as Google and Netflix use algorithms to predict and display what will be most useful for the user. Slavin worries humans are programming computers to run elaborate algorithms, but then the computer sort of takes over and then humans are left not fully understanding what is going on. For example, a majority of trading in the stock market occurs via algorithms because computers are much more efficient at predicting stock prices and making trades. However once these algorithms are placed in action, the computer begins making trades meanwhile humans may have no idea what is going on behind the scenes. In 2010, this proved to be problematic because 9% of US stocks disappeared due to algorithms and no body has any idea why.
Slavin also expresses worry that using such powerful algorithms will create a “monoculture”. Take Netflix, for example, which offers suggestion of what to watch next based on the movies or shows that you have previously watched. The algorithm works a bit differently than most people would expect because rather than just matching the genre of the movie, it matches various attributes that the movie has and suggests movies that have similar attributes (Abu-Mostafa). Slavin’s worry is that “the algorithm is taking ideas about human behavior and coding them and reinforcing them” (Slavin). In other words, the Netflix algorithms is essentially choosing what movies our culture will watch creating this idea of a monoculture.
 While disappearing stock and monoculture are definitely valid worries to have, algorithms have also allowed humans to do some pretty cool things. Take Watson, the Jeopardy! computer built by IBM, which is able to beat Jeopardy’s grand champions using algorithms and an enormous database. Watson is able to compete using four different strategies throughout gameplay. First, strategizes a wager for Daily Doubles, second, it strategizes a wager during Final Jeopardy, third, it determines which question to choose next, and fourth, it decides when to attempt to answer (Tesauro, G., et al.). Using these four algorithms, Watson is able to simulate a human mind and even beat human opponents.
While disappearing stock and monoculture are definitely valid worries to have, algorithms have also allowed humans to do some pretty cool things. Take Watson, the Jeopardy! computer built by IBM, which is able to beat Jeopardy’s grand champions using algorithms and an enormous database. Watson is able to compete using four different strategies throughout gameplay. First, strategizes a wager for Daily Doubles, second, it strategizes a wager during Final Jeopardy, third, it determines which question to choose next, and fourth, it decides when to attempt to answer (Tesauro, G., et al.). Using these four algorithms, Watson is able to simulate a human mind and even beat human opponents.
Watson marks a major milestone in computer science in that it able to use artificial intelligence in a more human way. Jeopardy clues are more involved than mere trivia questions, not only because players are given an answer rather than a question, but also because the player needs to have a good grasp on the English language since some clues involve homophones or a play on words. In addition, Watson is able to determine how to effectively wager. Computer scientists have had trouble replicating this type of thinking in a machine in the past, which makes Watson a huge achievement in the field (Tesauro, G., et al.).
One worry related to Slavin’s talk is that computers like Watson will be able to replace humans some day. Similar to problems in the stock market, this would be problematic since in some cases, even Watson’s creators don’t even fully understand how Watson computes an answer. For example, one clue was “Its largest airport is named for a World War II hero; its second largest for a World War II battle” and Watson responded with “what is Toronto?” (Markoff). So, while it’s amazing how far humans have come in computer science, it seems that the important thing is to make sure that we really understand the technologies that we are using.
Sources:
Abu-Mostafa, Yaser S. “Machines That Think For Themselves.” Scientific American 307.1 (2012): 78-81. Academic Search Complete. Web. 31 Mar. 2015.
Markoff, John. “Computer Wins on ‘Jeopardy!’: Trivial, It’s Not.” New York Times 16 Feb. 2011. Web
Slavin, Kevin. “Welcome To The ‘Algoworld’.” New Scientist 211.2826 (2011): 28-29. Academic Search Complete. Web. 31 Mar. 2015.
Tesauro, G., et al. “Simulation, Learning, And Optimization Techniques In Watson’s Game Strategies.” IBM Journal Of Research & Development 56.3/4 (2012): 16:1-16:11. Academic Search Complete. Web. 10 Apr. 2015.
~
The Expansive Possibilities of Teaching Statistics Before Calculus
Guest Author: Jeff Prior
In Arthur Benjamin’s TED talk segment “Teach statistics before calculus!” he calls for the need to change the pinnacle of education in mathematics. He argues that under the current education system, as students enter undergraduate study, the summit of the curriculum is calculus. He goes on to explain the importance of completing calculus within a student’s freshman year of college if that is what their field of study calls for (majors in math, physics, and economics). Benjamin is not discounting the necessity of calculus in these fields, however he is asserting that calculus is not usable on a day-to-day basis for the majority of students.
Statistics on the other hand is the math of analyzing any sort of data. Whether it is in the fields of math and science, or even in business, marketing, and psychology, statistics are much more than a study solely for mathematicians. After all, statistics is the “mathematics of games, and gambling, its analyzing trends, predicting the future.”
Although he does not go into a great deal of depth on the topic, he hints at the potential for students to expand statistics into their own interests. It is not his argument that calculus cannot be applied to these interests. Rather, his argument stresses that statistics are more valuable for most students in understanding and predicting the outcomes of there interests on an everyday, real world basis.
At Seton Hall University, a precedent is currently being set for schools to teach statistics before calculus. Instead of jumping straight into calculus for students in the math department, they now have the opportunity to take a game of their interest and look at it in a statistical manner. This way they can develop a better understanding and predict the outcomes of this game. This game is the game of baseball. The course at Seton Hall focuses on teaching the application of statistics to Major League Baseball and more specifically, new statistical methods in the game called Sabermetrics (Costa).
The study of Sabermetrics began in 1971 with the founding of the Society for American Baseball Research. Pioneer Bill James led the research, and in turn, a new form of baseball statistics were born. Sabermetrics are new measures of a player’s performance over their careers. Using these numbers, teams can predict how players will perform in the future and in certain situations, thus giving insight to weaknesses to opposing teams along with to the general manager of teams interested in said player to know if the player is worth their valuable investment. The most popular sabermetric statistical categories are runs created, power factor, modified offensive average, and total average.
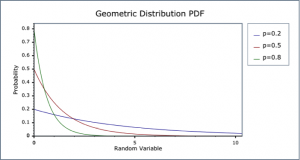
All sabermetric categories are based upon very simple statistical concepts. Most categories use linear weights and expected values. However, some of these stats are based upon estimations considering quasigeometric distributions. This is a distribution that is geometric at every point except for the starting point. This distribution is then used to weight current statistics used in baseball such as batting average, on base percentage, and slugging percentage. By multiplying these “standard” statistics and weighting them according to the distribution, they can predict how and when players will perform their best and worst. The quasigeometric distribution is a very good model for the estimation of when runs will be scored in a particular baseball game as shown in the figure below (Glass).
 Here we can see how in general, the predictive statistics above follow the standard geometric distributions below (with different p values not as important for this discussion).
Here we can see how in general, the predictive statistics above follow the standard geometric distributions below (with different p values not as important for this discussion).
Thus, the distribution is used to formulate predictions of specific players performances not only over the course of a game, but also a season, and a career. Some of the general formulas generated for Sabermetrics are shown below (Costa).
RUNS CREATED = (H + W + HP-CS) (TB + .65[SB + SH + SF]) /(AB+W+SH+SF+CS+HP+GIDP)
MODIFIED OFFENSIVE AVERAGE = (TB+SB+W+HP) / (AB+W+HP)
TOTAL AVERAGE = (TB + W + HP + SB) / (AB-H + CS + GIDP)
If teams have the ability to predict a player’s performance over their career, it increases their probability of making more reasonable decisions in paying the player, along with the probability of finding a way to defeat the player when they are in opposition.
By teaching a mathematics course on these Sabermetrics at Seton Hall University, they are following the path that Arthur Benjamin laid out in his talk. This school has successfully demonstrated how statistics can be more useful than calculus for the general student to understand and analyze the trends of their interests. Thus, the faculty at Seton Hall seems to have supported the call for the consideration of teaching statistics before students embark in calculus.
Sources:
Benjamin, Arthur. Teach statistics before calculus! TED, 2009. Web. 31 Mar. 2015.
Costa, Gabriel B. “Baseball In The University Classroom: Sabermetrics.” Education 112.2 (1991): 273. Academic Search Complete. Web. 28 Apr. 2015.
Glass, Darren. “Quasigeometric Distributions and Extra Inning Baseball Games.” 81.2 (2008). JSTOR. Web. 31 Mar. 2015.
~
Fibonacci Numbers and Teaching Math as Art
Guest Author: Jessica Launius
According to Arthur Benjamin, there are three reasons math is studied: calculation, application, and inspiration. Unfortunately, it is the third of these reasons that is often forgotten and overlooked. In his Ted Talk titled, “The Magic of Fibonacci Numbers,” Benjamin explores the beauty hidden in Fibonacci numbers and illustrates one example of being inspired by mathematics.
His exploration of the Fibonacci numbers focuses on the patterns that exist within the Fibonacci numbers themselves. He illustrates first for the audience that the sum of the squares of n Fibonacci numbers is equal to the product of the nth Fibonacci number and the previous one.
 However, his talk goes beyond showing this phenomenon to explaining why this should happen, and part of the magic of this talk is his use of a straightforward, visual proof. He proves this to be true by constructing a rectangle formed by the squares of the Fibonacci numbers, and calculates the area of that rectangle in two distinct ways: summing the areas of the individual rectangles, and multiplying the base and the height of the whole rectangle. Because both methods for calculating area are valid, the sum of the squares must be equal to the product of the last two numbers in the sequence.
However, his talk goes beyond showing this phenomenon to explaining why this should happen, and part of the magic of this talk is his use of a straightforward, visual proof. He proves this to be true by constructing a rectangle formed by the squares of the Fibonacci numbers, and calculates the area of that rectangle in two distinct ways: summing the areas of the individual rectangles, and multiplying the base and the height of the whole rectangle. Because both methods for calculating area are valid, the sum of the squares must be equal to the product of the last two numbers in the sequence.
By demonstrating this proof in a tangible way, Benjamin effectively engages his audience in the beauty to be found in the Fibonacci numbers, thus showing them that math can be studied for inspiration. Unfortunately, this reason for studying mathematics is one that is often overlooked in high school classrooms.
A separate study by Chronaki explores the implementation of teaching math as an aspect of culture, and expresses concerns about the “learning for practicality” approach often used when teaching mathematics, particularly in the way of stifling students’ creative processes. When studying classrooms in which teachers talk math as something to be appreciated and explored, Chronaki found these classrooms had a high level of student engagement.
As it seems clear there are benefits to teaching students math for the use of inspiration, the question then remains of how teachers can do this. Chronaki, through his conversations with teachers, makes it clear this is not an easy task, as teachers are used to the traditional model of teaching as acquisition of knowledge.
We must ask ourselves if this challenge is not made more difficult by the current standards for education, and the American insistence on standardized testing. Furthermore, we must wonder whether or not the math currently taught in high school lends itself to beauty. An essay by Rota attempts to break down what it means for mathematics to be “beautiful” and provides a lens for this difficult task.
Beauty in mathematics, according to Rota, can come in a variety of forms, and relates to how the math is presented. Clever steps in proofs, theorems that capture what is inherently held to be true, and ideas or patterns that surprise and astound fall into this category of beauty. Though Rota admits these illustrations of beauty are objective, his suggestions, particularly in regards to looking for something that is surprising, can provide a spring board for moving forward and determining where beauty already exists in the curriculum in order to expand on those areas.
Though teaching students a rigorous proof with one interesting step may not be enough to convince them math is beautiful, continued glimpses into math as surprising, tangible, and interesting can aid in teaching students that math is not simply practical application, but also beauty to be inspired by.
It’s said by any number of math teachers: math is everywhere. Yet students protest because the math they view in the classroom is not the math they encounter in their lives, and not the math that they can be inspired by. Making a move towards teaching math as art and revealing this beauty to students offers a way to change the perspective of the coming generation, which, given time, can lead to reform as whole.
Sources:
“Teaching Maths through Theme-Based Resources: Pedagogic Style, ‘Theme’ and ‘Maths’ in Lessons.” A. Chronaki. Educational Studies in Mathematics. Vol. 42, No. 2 (2000), pp. 141-163
“The Phenomenology of Mathematical Beauty.” Gian-Carlo Rota. Synthese. Vol. 111, No. 2, Proof and Progress in Mathematics (May, 1997), pp. 171-182
Photo Source: TED.com
~
Using Mathematics to Generate Music
Guest Author: Kelly McCurry
In Natalya St. Clair’s video, Music and math: The Genius of Beethoven, ideas of using math to explain music was addressed through the example of Beethoven composing music using some ideas founded in math since he slowly went deaf over the last half of his life. Did Beethoven really use math to compose? While this answer is truly unknown, a post reflecting on the same video focused around this question can be found here. Instead I am going to focus on the relationship between math and music. This idea has been around for a long time, but more recently through math and physics. People have been trying to understand the harmonic structures of sound and use their discoveries to help develop a way for computers to produce sounds that are almost identical to everyday sounds.
The main challenge that occurs with reproducing sounds is reproducing the exact same sound waves. When most people think of sound waves, they picture the shape of a vibrating string after it has been plucked, which is approximately a sine curve. This is a type of wave, but only represents a single frequency. This is very different from the world we know, because all of our sounds consist of a fundamental frequency, the lowest and loudest tone heard, and what are called harmonics. Harmonics are integer multiples of the fundamental frequency, or could be called octaves above the fundamental frequency. All of these sounds when looked at individually are sine curves. When people tried to generate sounds on a computer, they focused on playing just the fundamental frequency, creating harsh versions of tones we hear in our everyday lives. This is the more simplified version of sound that is usually discussed in school, and was discussed in the video and it is an approach that does not work with the desire to generate music using computers.
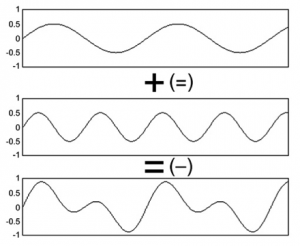
So instead we have to look at all of the waves that generate the sound and the harmonics. These types of waves come in three main types: saw tooth, square and triangle. These curves are not just composed of one sine curve but multiples put together to generate a new curve. The following is a simple example of how two curves can be added together to make a new one.
But in reality, every sound is generated by infinitely many waves added together, ones we can hear and others we cannot, but together they can be generated by the Fourier series. Like the sine curves that help to form the series, the series itself generates a periodic wave, most traditionally the three discussed above. The Fourier series is the infinite sum of sinusoidal function as follows:
We can write waves using this formula, but actually any periodic function can be written using the Fourier series. When looking at the formula above, we can see the different harmonics being included in the series since the n in both summations represents the integer multiples of the fundamental frequency. For more information as to how this formula for this use was derived, reference [4]. In the late 1990’s, this was used to generate electronic music on computers that was beyond the single pitch sounds traditional for the times, helping to move the industry beyond sounds that were not pleasing to listen to. You can see some of this work here, and while it is more advanced it is still unpleasing to listen to for long period of time.
Using this formula, Neuwirth was looking at specific sinusoidal functions that had many of the characteristics of sound waves, such as having a minimum and maximum along with 3 turning points in a single period. Why are these characteristics important to making a sound wave? Most of the curves that he found were halfway symmetric with itself during a single period. While he was able to generate a variety of curves, he still found them to be not pleasing to the human ear. A single wave at the time was found that was not halfway symmetric that sounded more pleasing to the ears. This wave is pictured below.
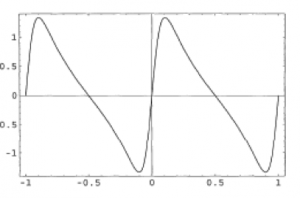
Neuwirth, Erich. “Designing A Pleasing Sound Mathematically.” Mathematics Magazine 74.2 (2001): 91. MasterFILE Premier. Web. 9 Apr. 2015.
This wave is found through the use of the Fourier series, and uses the geometric series generated by ½ as the coefficients. This was the start of the advances in electronic music and pitch generation and shows the complexity sound waves have within our world.
Since the Fourier series’ sounds are generated electronically, a new problem has arisen. The sounds are not truly themselves because they are being generated by the computer not the way nature makes the sounds, which causes distortion in a way that sounds become indistinguishable when played together, a term known as aliasing distortion. This is the next step in being able to use computers to generate accurate and pleasing sounds, comparable to the sounds in nature. With these new advances, will we be able to generate sounds that sound like nature or should we leave it up to Mother Nature and musicians to amaze us with beautiful harmonies and melodies?
Sources:
Music and Computers: A Theoretical and Historical Approach. 27 Apr 2015
Music and math: The Genius of Beethoven – Murray Bourne. 27 Apr 2015
Music and math: The Genius of Beethoven – Natalya St. Clair. 27 Apr 2015
Neuwirth, Erich. “Designing A Pleasing Sound Mathematically.” Mathematics Magazine2 (2001): 91. MasterFILE Premier. Web. 9 Apr. 2015.
Petersen, Mark R. “Musical Analysis And Synthesis In Matlab.” College Mathematics Journal5 (2004): 396-401. General Science Abstracts (H.W. Wilson). Web. 9 Apr. 2015.
Realtime Fourier synthesis – Sound generation 27 Apr 2015
Schimmel, Jiri. “Audible Aliasing Distortion In Digital Audio Synthesis.” Radioengineering1 (2012): 56-62. Computers & Applied Sciences Complete. Web. 9 Apr. 2015.
~
Why Mathematics Instruction IS Necessary
Guest Author: Kimmy Grimmer
 The talk “Why Math Instruction is Unnecessary” caught my attention just by the title (link below). John Bennett, a middle school and high school math teacher, claims that upper level math courses in high school should not be required for students. His claim is based off of students always asking “When are we going to use this in real life?” Bennett has tried multiple ways to engage his students in mathematics instruction by trying to show that math is everywhere, helpful, and cool, however that didn’t work for most of his students. So he tried showing his students their options if they take upper level math classes such as becoming an engineer, physicist, etc. He counters his own claim by realizing that only .5% of the population are engineers and even if you double that percentage, only one percent of the population uses higher math. Finally his last approach was to encourage students to get good grades, go to a good college, and get a good grades. But that didn’t seem to sit right with him nor does it for me. Bennett did not want to use that as his reasons for encouraging students to be engaged especially since higher SAT scores didn’t necessarily lead to being more successful later in life. Lastly, he explained how he talked to his students and some found that math was scary. Bennett says, “Kids really enjoyed math until (insert bad math teacher’s name) turned them away from the subject” (“Why Math Instruction Is Unnecessary”). People, students and parents alike, have math anxiety if you can even believe that’s a real thing. Bennett’s conclusion from his talk is most people learn the amount of math they will need to know for their lives by the time they are 10 and we shouldn’t force students who don’t want to take math and have math anxiety to take upper level math courses. However, John Bennett explained that we should introduce brain games that stimulate the type of analytical thinking students will need to do when they graduate high school.
The talk “Why Math Instruction is Unnecessary” caught my attention just by the title (link below). John Bennett, a middle school and high school math teacher, claims that upper level math courses in high school should not be required for students. His claim is based off of students always asking “When are we going to use this in real life?” Bennett has tried multiple ways to engage his students in mathematics instruction by trying to show that math is everywhere, helpful, and cool, however that didn’t work for most of his students. So he tried showing his students their options if they take upper level math classes such as becoming an engineer, physicist, etc. He counters his own claim by realizing that only .5% of the population are engineers and even if you double that percentage, only one percent of the population uses higher math. Finally his last approach was to encourage students to get good grades, go to a good college, and get a good grades. But that didn’t seem to sit right with him nor does it for me. Bennett did not want to use that as his reasons for encouraging students to be engaged especially since higher SAT scores didn’t necessarily lead to being more successful later in life. Lastly, he explained how he talked to his students and some found that math was scary. Bennett says, “Kids really enjoyed math until (insert bad math teacher’s name) turned them away from the subject” (“Why Math Instruction Is Unnecessary”). People, students and parents alike, have math anxiety if you can even believe that’s a real thing. Bennett’s conclusion from his talk is most people learn the amount of math they will need to know for their lives by the time they are 10 and we shouldn’t force students who don’t want to take math and have math anxiety to take upper level math courses. However, John Bennett explained that we should introduce brain games that stimulate the type of analytical thinking students will need to do when they graduate high school.
Now as an aspiring mathematics teacher I couldn’t believe that someone who is currently a math teacher would say that math instruction is unnecessary. However, after listening to Bennett’s claim and trying to understand how he could make such an extreme statement I understood his reasoning behind it. In fact, I was on the bus with the soccer team I coach and found that half of my athletes have what is called math anxiety. That just didn’t sit right for me, but I knew that Bennett had made a great argument based off of the current style of math instruction in high schools. Not all students are going to need higher level math instruction in high school. Some will never use anything past algebra in their careers, so why do they have to take it? Bennett argues that there is no point in students taking such specific higher level math courses. As a math teacher, I have struggled with this dilemma as well. I taught Algebra II and many of my students did not see the point in the course because they were never going to use it. It’s possible some of my students would benefit more from the “brain games” that Bennett talks about. So how do we go about promoting both and helping all students gain the math instruction they will need in the future. I looked further into why mathematics instruction is important, how we can make it better, and how to prevent and cure math anxiety.
The article “K-12 Science, Technology, Engineering, And Math (STEM) Education For America’s Future” stated that STEM instruction is vital because “it will generate the scientists, technologists, engineers and mathematicians who will create new ideas products and industries of the 21st century” (1). That seems like one of the strongest reasons why mathematics instruction is indeed necessary in middle and high school for some students. Mathematics instruction also helps our problem solving ability as well as our decision making ability to make sure that as citizens we are all making informed choices. Students need to be provided the proper instruction in order to be able to learn and use this skills after high school. One problem with math instruction is that most schools lack the teachers who love their subject and want to share that love with their students and be able to teach their subject effectively. This is one of the reasons why Bennett’s claim holds true. Another reason that math instruction seems unnecessary is that students decide early on that they do not like math is because it’s boring, too hard, or unwelcoming (“K-12 Science, Technology, Engineering, And Math (STEM) Education For America’s Future”, 1). One possible solution to the problem would be to have strictly STEM schools for students wanting to pursue those subjects. I think Bennett would support STEM schools for students truly wanting to pursue a career using higher level math.
Another issue that comes to mind when hearing that one idea is to eliminate higher level math courses for students who do not want to pursue a career in mathematics is that most students in high school do not know what they want to do with their lives when they graduate high school and college. Students need the exposure to the different levels of math to prepare them for possible careers that might need calculus and trigonometry whether they know they want to pursue those careers yet or not. In fact it was found that the percentage of students entering STEM subjects in college was higher if they took those upper level math courses in high school (Chen, 7). We want students to pursue careers in STEM subjects to keep the U.S. competitive in innovation and technology so we need to expose them to the types of math they might want to use or need to use in the future.
The last claim of Bennett is that in a mathematics classroom teachers should be doing brain games and activities that stimulate the type of thinking that students will need after high school that are not specific to math content. He makes a great point when he says this because students could benefit from this type of brain stimulation that really gets them logically thinking about the subject. So the final question I pose is what should students be doing in a math classroom? Is it truly the content that is vital for everyone or do we just need to provide students a safe learning environment to develop the deductive and inductive reasoning skills they will need. My answer is both. For some students they will need the content and others might just need the skills, however, as math instructors we can teach students the thinking skills they will need through the upper level content (Fumer & Berman, 171). Mathematics teachers need to start by fixing the notion of math anxiety, supporting all of their students, and ensuring that students achieve success in mathematics to prevent people from thinking mathematics instruction is unnecessary in high school.
Sources:
Bennett, John. “Why Math Instruction is Unnecessary.” YouTube. YouTube, 7 Nov. 2011. Web. 12 Apr. 2015.
Chen, Xianglei. “Students Who Study Science, Technology, Engineering, and Mathematics (STEM) in Postsecondary Education. “ National Center for Education Statistics.(2009). Web. 2 Apr 2015. http://nces.ed.gov/pubs2009/2009161.pdf
“K-12 Science, Technology, Engineering, And Math (STEM) Education For America’s Future.” Tech Directions 70.6 (2011): 33-36. Academic Search Complete. Web. 2 Apr. 2015.
Fumer, Joseph M. & Barbara T. Berman. “Math Anxiety: Overcoming a Major Obstacle to the
Improvement of Student Math Performance.” Childhood Education 79:3 (2003): 170-17. Web. 10 Apr 2015. http://dx.doi.org/10.180/00094506.2003.10522220
~
From Sonars to Music to Cryptography
Guest Author: Lauren Carlson
True randomness is one of the hardest things for the human mind to do, almost to the point where it is impossible. The human mind has evolved to find patterns and predict what the most likely outcome is. This is an essential skill to survive and is also shown in many animals. In another TED talk, argues that intelligence is truly measured by the ability to detect patterns in order to predict possible future events.
Sonars have become the essential key to navigation in submarines. During WWII, submarines became a large part of the battle and their navigation techniques needed to be advanced. Sonars emit a sound wave at a certain frequency and measure how long it takes that frequence to return to the receiver. Using a set on physic equations, we can find how far an object is and how fast it is moving. A sonar normally emits multiple sound waves to lay a map for the surrounding environment as a submarine is navigating.
In order to make the sonar as accurate as possible a random generation of frequencies is needed. This set of frequencies has no common interval between any two consecutive elements which leads to no repetition. This was accomplished by using Costas Arrays which were developed by Costas using Galois number theory. Using modular arithmetic Costas created the equation 3x mod 88. This then generated the set needed to more effectively use in sonars.
For this talk, mod 88 was used because there are 88 notes on a piano. In the future we may be able to increase that number as sonar receivers become more sophisticated and accurate. Galois Theory has many other applications in the more recent past. The theory is used in cryptography and many types of technological encoding. Without Galois theory we would not have out smart phones or computers at the level that they have reached.
Another application of Costas arrays is found in the field of cryptography. Costas arrays can be used to communicate messages using a key. Because these numbers are pseudorandomly generated it is almost impossible to find the correct sequence within the lifespan of an average human, at least with where computer science is presently. These applications can be used in banking, politics, war zones, etc. This shows how a mathematical idea can be transferred from one area to another.

http://news.sciencemag.org/sites/default/files/styles/thumb_article_l/public/media/sn-cryptography_0.jpg?itok=a4H3ZoHu
Sources:
DRAKAKIS, K. (2009). A REVIEW OF COSTAS ARRAYS. Journal Of Applied Mathematics, 1-32. doi:10.1155/JAM/2006/26385. (2012) McGraw-Hill encyclopedia of science & technology.New York : McGraw-Hill,
Meijer, A. (1996). Groups, factoring, and cryptography. Mathematics Magazine, 69(2), 103.
~
Classroom Makeover
Guest Author: Mike Hanson
In the TED talk “Math Class Needs A Makeover”, Dan Meyer explains why math education in the U.S. today is corrupting students. He classifies two types of solving math problems. The first type is Computation, and the second is Math Reasoning. Meyer focuses on teaching Math Reasoning to his students, as he believes this is the type of math that appears in real life applications. Typically, a mathematics textbook teaches Math Reasoning to students in a way that they won’t remember it. Students find themselves eagerly looking for a formula to easily solve a word problem. As a result, they become impatient with any problem that can’t be resolved quickly. Most problems are worded in a way that gives the student all of the information needed to plug into a formula. This doesn’t allow the student to get fully engaged in understanding the problem.
Meyer believes that involving students in the formulation of a problem is the best way to get them engaged, and ultimately seeing the usefulness of math in the real world. The example he gives is a word problem about how long it would take to fill a water tank.
As you can, all of the information to solve this problem is given, and is just waiting for the student to plug in the variables to a formula. Meyer changes this problem to give as little information as possible.
He then shows his class a video of filling up a similar tank and gets the students to have a conversation about how they are going to solve this problem. This conversation is what Meyer refers to “baiting the hook”, or intriguing his students in the problem. By doing this, Meyer is making the students decide what matters in the problem, which is essentially putting all of the students on a level playing field of intuition. By letting the students build the problem, they become engaged in solving it.
In “Students’ Construction of Formulas in Context” by Martin Van Reeuwijk and Monica Wijers, a math curriculum is described that focuses on the construction of formulas by the students. They argue that algebra is a tool for making sense of the world. By motivating students to use their own strategies to solve these problems, they are able to connect to them in a meaningful way. This curriculum lets the students explore the problem and understand what it is about. The purpose of keeping the problem open is to avoid the students from using a given formula, and forcing them to further explore the problem on their own. In this process, the role of the teacher becomes just a guide, allowing the students to do most of the learning on their own.
An article published in the New York Times entitled “How to Fix Our Math Education”, by Sol Garfunkel and David Mumford, expresses the concern about poor math education in the United States. It argues that a curriculum focused on real-life applications would be best for students. These types of problems presented to the student will help them appreciate how to use mathematical formulas for useful situations. As a result, it would intrigue them to develop an answer on their own, and not simply plugging numbers into a formula. With this type of learning approach, students may more easily retain mathematics, and also find usefulness of mathematics in the real world.
The next time you find yourself leading a math talk or even being a part of the audience, I challenge you to think about how the content is being presented. Think about the possible ways to engage the audience and hook them into the topic. By doing this, you will have created intuition about the information in front of them. This intuition can help them formulate their own solutions and truly understand the usefulness of mathematics.
Sources:
Garfunkel, Sol and Mumford, David. “How to Fix Our Math Education.” The New York Times. 24 Aug. 2011. http://www.nytimes.com/2011/08/25/opinion/how-to-fix-our-math-education.html?_r=0.
Meyer, Dan. “Math Class Needs a Makeover.” TED. Mar. 2010. http://www.ted.com/talks/dan_meyer_math_curriculum_makeover?language=en.
Van Reeuwijk, Martin and Wijers, Monica. “Students’ Construction of Formulas in Context.” JSTOR. Feb. 1997.
~
Models to Represent Hyperbolic Geometry
Guest Author: Paul Landahl
When introducing students to the concept of hyperbolic space, mathematicians are limited. Some physicists say that the shape of the universe is hyperbolic. I’m sure you have seen a demonstration in the classroom, on YouTube or even on some PBS space special of a bowling ball on a stretched on fabric. The heavy object creates a bend in the sheet, representing a celestial body creating a bend in space-time via gravity. This image, made famous by scientists, is a convenient image for the shape of hyperbolic space, for many people.
Mathematicians have often relied on a different model to show hyperbolic space. In 1871, French mathematician Henri Poincaré mapped the hyperbolic plane to a disk without changing angles. The Poincaré model was made popular beyond the math realm when it was adopted by artist M.C. Escher.[1] Today there are tools that students can use to explore hyperbolic space and the Poincaré model.[2]
However, the mathematical model seems to be lacking something compared to the physical model. Dr. William Thurston believes that many people have a poor impression of mathematics relying complicated and confusing rules.[3] This is not a natural phenomenon, but rather a consequence of how the material is presented through years of schooling. While traditional math models can be tedious and formal, the computer animated model on NOVA has charisma.
After reading excerpts from Daina Taimina’s book Crocheting Adventures with Hyperbolic Planes and watching her TED talk about the same topic, I began to realize that mathematical representations of hyperbolic space can be presented in a much more accessible method: the art of human understanding.[4]

http://chronicle.cornell.edu/stories/2005/12/crocheting-curved-space-illustrate-indescribable-geometry
To break this barrier, Dr. Taimina began crocheting hyperbolic plans, using a variance of colors, sizes, and designs, bringing to life this complicated mathematical idea. Using the crochet models, we can interact and see straight lines, radii, and circumference on the hyperbolic plane. [5]
The benefit of Taimina’s model is that it doesn’t begin with rigor and rules. The crochet planes “are very inviting” and encourage “endless possibilities for play.”[6] Students will then be inclined to ask their own questions about hyperbolic space, leading to questions about curvature, manifolds, shape, sides, lines and
points. Natural inquiry questions begin to arise, like “how does curvature behave when we flatten them?” Hyperbolic space is an inherently fascinating subject, so why not make it fascinating for the students?
Sources:
[1] Douglas Dunham, “M.C. Escher’s Use of the Poincaré Models of Hyperbolic Geometry,” http://www.math-art.eu/Documents/pdfs/Dunham.pdf.
[2]Daina Taimina, Crocheting Adventures with Hyperbolic Planes, (Wellesley, MA: A.K. Peters, 2009), 2-4. Personally, I like to use a java applet of an interactive Poincare model. A version of the applet can be found here: https://www.cs.unm.edu/~joel/NonEuclid/NonEuclid.html
[3]Ibid., ix.
[4] You can find Dr.Taimina’s TED talk here: https://www.youtube.com/watch?v=w1TBZhd-sN0
[5] Taimina, “What Is the Hyperbolic Plane? Can We Crochet It?” In Crocheting Adventures with Hyperbolic Planes.
[6] Ibid., 87.
~
Symmetry in Science
Guest Author: Rebecca Post
Symmetry is often thought about as a simple mathematical concept. Something is symmetrical if you can draw a line through it and one side is the mirror image of the other. Symmetry is more than that though, as Marcus du Sautoy describes in his talk “Symmetry, reality’s riddle.” The symmetries explored in mathematics, specifically abstract algebra, deal with how the different symmetries of an object interact. One of the examples du Sautoy gives is of artwork in the Alhambra in Granada. The designs pictured below look completely different, but the ways the designs rotate to produce an image that looks unchanged are the same, meaning they both are representations of the same symmetrical object.
 Symmetry does not only occur in artwork, but naturally in the world around us as well. Du Sautoy described symmetry as “nature’s language” and with good reason. The symmetries in the natural world give us insight into the objects. One of the most basic examples of a symmetric object in the natural world is a snowflake. A snowflake can be rotated or reflected in many ways resulting in the snowflake looking no different than before the rotation or reflection.
Symmetry does not only occur in artwork, but naturally in the world around us as well. Du Sautoy described symmetry as “nature’s language” and with good reason. The symmetries in the natural world give us insight into the objects. One of the most basic examples of a symmetric object in the natural world is a snowflake. A snowflake can be rotated or reflected in many ways resulting in the snowflake looking no different than before the rotation or reflection.
 Mathematics’ connection to physics is typically tied to the derivation of equations to make predictions and typically involve calculus or similar areas of mathematics. Symmetry though has proven to be important to physics in the field of string theory. Theorists believe that the universe was formed by the breaking of internal symmetries. Scientists are experimenting on this theory by trying to break symmetries in liquid crystals. In addition, in the theory called “Supersymmetry” for every boson there is a corresponding fermion (bosons and fermions are different particles) and these particles are connected. While the existence of these particles from Supersymmetry simplifies equations in string theory, they still have not been proven to exist. Symmetry though has become an important part of the theory because symmetry is so inherent in nature, that symmetry in the theory of everything seems only natural.
Mathematics’ connection to physics is typically tied to the derivation of equations to make predictions and typically involve calculus or similar areas of mathematics. Symmetry though has proven to be important to physics in the field of string theory. Theorists believe that the universe was formed by the breaking of internal symmetries. Scientists are experimenting on this theory by trying to break symmetries in liquid crystals. In addition, in the theory called “Supersymmetry” for every boson there is a corresponding fermion (bosons and fermions are different particles) and these particles are connected. While the existence of these particles from Supersymmetry simplifies equations in string theory, they still have not been proven to exist. Symmetry though has become an important part of the theory because symmetry is so inherent in nature, that symmetry in the theory of everything seems only natural.
Physics though is not the only science that is connected to mathematics. Biology has also found a need for mathematics, specifically the symmetries mathematics helps to understand and uncover. For example, mathematics has actually played a large role in our understanding of viruses. Viruses take on complex shapes such as the icosahedron, which has been shown to have 120 different symmetries (rotations or reflections). One of the ways scientists are working to develop cures is by analyzing the symmetries. For example, researchers have noted that viruses that typically take the shape of an icosahedron are not infectious when shaped like a tube, meaning if the researchers could interfere with the symmetry of the object by producing the proteins that lead to the tubular shape, the virus could be stopped.
 Symmetry is becoming more and more important to the different sciences, showing that applications of abstract algebra and group theory exist though those working in abstract algebra tend to focus on further abstraction rather than application. It is important to realize that these abstract mathematical ideas can be applied to the sciences, to help look at phenomena in a different light.
Symmetry is becoming more and more important to the different sciences, showing that applications of abstract algebra and group theory exist though those working in abstract algebra tend to focus on further abstraction rather than application. It is important to realize that these abstract mathematical ideas can be applied to the sciences, to help look at phenomena in a different light.
Sources:
Du Sautoy, M. (2008). Grand designs. New Scientis, 198(2660), 38-41.
Peterson, I. (1991). Cracks in the cosmos. Science News, 139(22), 344.
Shea, C. (2013). Is scientific truth always beautiful?. Chronicle of Higher Education, B14-B15.
Stewart, I. (2011). Fearful symmetry. New Statesman, 140(5050), 38-41.
http://www.ted.com/talks/marcus_du_sautoy_symmetry_reality_s_riddle?language=en
http://scienceblogs.com/photosynthesis/2010/01/05/snowflakes/
http://math.wikia.com/wiki/Icosahedron
~
High School Math Focus: Calculus or Statistics?
Guest Author: Rebecca Sund
Arthur Benjamin discusses that mathematics curriculum at the high school level is built upon a foundation of arithmetic and algebra with the summit of the high school math pyramid being Calculus. However, Benjamin argues that a more applicable summit would be probability and statistics. But before getting into this, let’s take a closer look at how math has gotten to where it is in high schools today. William Berlinghoff and Fernando Gouvea discuss how Galileo and Descartes generated questions about the universe that could only be answered through mathematical ideas now described as Calculus. They describe the work of Leibniz and Newton led to the creation of books and the use of calculus by mathematicians to study the natural world (p. 42-43).
Irving Cowle takes this a step further and discusses a particular event in the 1950’s that helped shape the role of mathematics in school. In 1957, the Russians launched Sputnik and America began to panic. He writes “The shock and amazement was national and the response was instantaneous. The cry was to do something and help us catch up to the Russians” (69). Sputnik created a national desire for more rigor in schools, more science based math applications and as a result, an emphasis was placed on calculus.
However Berlinghoff and Gouvea also pose a question that aligns very closely with Arthur Benjamin’s ideas. To paraphrase; Should mathematical educators allow internal demands of the subject to dictate rigorous training in traditional areas so that the future generations will have a firm foundation for their learning, or should they let the external needs of society dictate a less intensive education that will allow everyone become mathematically literate citizens? (p. 60). Should we change our curriculum in high schools to be more inclusive of real world applications, even if it means decreasing the rigor and prestige of the mathematical concepts taught?
Before answering this question, let’s take a closer look at why calculus is being viewed as an internal demand and what society is now demanding of its citizens in terms of mathematical knowledge. Calculus definitely has real world applications, from engineering to the laws of nature. So why is it now seemingly something only used within the walls of a math classroom? Arthur Benjamin also agrees that calculus has real world applications, but argues that it is not something that people use in their day to day lives, whereas probability and statistics can and should be in everyday life. George Grossman makes a compelling argument for making probability and statistics a priority in high school. He discusses that we are living in a world that is full of verbal and graphic statistical representations and writes that we “owe it to youth to prepare them for able analysis” (p. 540).The world we live in is a statistics driven world and without educating our high school students, we are potentially depriving them of the ability to be successful.
However, Grossman also recognizes the potential consequences of changing our high school curriculum. He argues that by incorporating statistics, we’d be excluding other topics, such as calculus (p. 541). Is it fair to replace the math that explains the laws of nature, is the basis for engineering and is the math of the ancient world with a newer math that explains gaming and gambling? Additionally, Arthur Benjamin never explains how the rest of the math curriculum would be structured, besides implying that calculus would be pushed back until college and does not discuss how this change would affect middle school math curriculum.
All in all, I think that there are very compelling arguments on both sides of this issue. However, I have obviously had experiences with high school curriculum and growing up in this digital world that Arthur Benjamin describes. I took AP Calc BC in high school and tested into Discrete Mathematics as a freshman at Augustana. I don’t think I would have chosen to study math in college and be graduating with a degree in mathematics if I had not had calculus in high school. But I also felt unprepared for probability and statistics classes since I had no exposure to these ideas in high school. Therefore, I think that stats should be included, but not sure about completely replacing calculus. I think that high school students should have the option to take one or the other or even both of these subjects. This would allow students to have control over their learning and use their high school math education to best suit their needs and prepare them not only to be successful in their profession, but also as informed and educated citizens.
Sources:
Benjamin, Arthur. “Teach Statistics Before Calculus.” TED. Feb. 2009. Lecture. http://www.ted.com/talks/arthur_benjamin_s_formula_for_changing_math_education?language=en
Berlinghoff, William P., and Fernando Q. Gouvea. “The History of Mathematics in a Large Nutshell.” Math Through the Ages: A Gentle History for Teachers and Others. Expanded ed. Washington, DC: Mathematical Association of America, 2004. Print.
Cowle, Irving. “Is the New Math Really Better?” The Arithmetic Teacher 21.1 (1974): 68-73.JSTOR. Web. 8 Apr. 2015. <http://www.jstor.org/discover/10.2307/41190813?uid=2134&uid=2&uid=70&uid=4&sid=21105959694471>.
Grossman, George. “Probability and Statistics in the Twelfth Year.” The Mathematics Teacher 54.7 (1961): 540-46. JSTOR. Web. 8 Apr. 2015. <http://fulla.augustana.edu:2071/stable/27956465?
~
Powerful Statistics…Powerful Applications
Guest Author: Reed Kottke
In Hans Rosling’s presentation, “The Best Stats You’ve Ever Seen”, the bounds of data collection crossed paths with the limits of data analysis and the pinnacle of data visualization. Using data from the preceding 40 years, models were created that clearly and concisely displayed the past and current status of various variables relating to world nations. These analysis’ were used to predict future activity, isolate variables that impacted well-being, recognize and measure events in history, and synthesize formulas that take into account sometimes forgotten variables. What Rosling did, in short, was display the capabilities of big data analysis.
Rosling’s talk focused on the change in economic and health states of nations around the world, all the while exposing misunderstood myths about the status of the world. However in a more broad sense, the mathematics of the talk displayed a process of capturing and storing data, then using the refined data to sequester trends and patterns, working backwards from these results to create real, proven formulas.  This “Big Data Analysis” is often simplified as such, as stated in “Big Data and Its Technical Challenges” ,“painstaking handcrafted models of reality … can now be made using data-driven mathematical models” (Jagadish, Gehrke, Shahabi, et al). In this particular case, the analysis was used to display global trends and predict future states, however the same methods are becoming the new age of statistical competitive advantages for businesses all over the world. By modeling and implementing the many variables involving a company such as customer experience, overhead cost, correlating profit (and many, many more, sometimes seemingly insignificant variables) that a business can gain this competitive advantage in many fields, as summarized in the Bell Labs Technical Journal (Spiess et al.).
This “Big Data Analysis” is often simplified as such, as stated in “Big Data and Its Technical Challenges” ,“painstaking handcrafted models of reality … can now be made using data-driven mathematical models” (Jagadish, Gehrke, Shahabi, et al). In this particular case, the analysis was used to display global trends and predict future states, however the same methods are becoming the new age of statistical competitive advantages for businesses all over the world. By modeling and implementing the many variables involving a company such as customer experience, overhead cost, correlating profit (and many, many more, sometimes seemingly insignificant variables) that a business can gain this competitive advantage in many fields, as summarized in the Bell Labs Technical Journal (Spiess et al.).
So what is Big Data? Jeffery Speiss (et. al.) classifies big data as having the three V’s: high Volume, high Velocity, and high Variety. The volume is obvious, these amounts of data are so large that typical database systems and mathematical algorithms are not capable of storing or interpreting them. This big data is too large and complex to capture, process and analyze using current computational infrastructure, thus creating a need for new systems and processes. The new infrastructure necessitates data structures beyond typical SQL and noSQL (not only SQL) databases, ushering in new forms of data structuring (Gudivada, V. N., Baeza-Yates, R., & Raghavan, V. V). The term “high velocity” refers to the constant stream of endless data which can be modeled and predicted infinitely. Lastly, the high variety refers to the various methods of capturing this data, from surveys to internet browsing capturing to analysis of existing data.
The real expertise in this data variety is knowing how each variable of initially unsorted data relates to other data, also referred to as “cleaning” the data. To some extent, this involves a brute force method of correlating data inputs to a single output. In Hans’ presentation, this was displayed when the child survival rate of a nation based upon time was compared to the GDP per capita by time. A steady correlation was found in each case. When  applied to such fields as business, two variables such as allocation of resources toward human resources and revenue stream can be modeled to find a very real and dependable correlation.
applied to such fields as business, two variables such as allocation of resources toward human resources and revenue stream can be modeled to find a very real and dependable correlation.
The sheer amount of data to be collected is massive. 90% of the world’s current data was created in the last two years (Jagadish, Gehrke, Shahabi, et al.). As such, collecting this data alone becomes a process. This breeds questions of privacy and ownership. Introducing the problem of data privacy, Jill Duffy comments that personal data may be considered intimate, and modeling of this data can sometimes yield unique and arguably private results. Consider an internet users browsing data triggering the attention of specific health care companies. Social data may be considered less private, however basic internet users may still want their everyday actions to go unstudied. So who owns this data: the collector of said data, the creator of the data, or the buyer of the data? Of course, this data is valuable in the right hands, and as such it is the very collection of data that has become an industry itself.
Once collected, the data must be formulated. The benefit of big data comes from skipping the process of creating and proving potential models. Hal Varian claims in “Big Data: New Tricks for Econometrics” that using large sets of data, a trend can be found and then continuously tested and confirmed using further collected data. Hypothetically, a data scientist would collect a multitude of data sets, all similar in that they are the results of the same phenomena. Now if say n sets of data are collected, data sets 1 to n-1 can be analyzed and a formula attained from their analysis. Now the nth can be predicted and then confirm the formula. Repeating this process continuously refines formula and guarantees the result. Potentially, these analysis’ can be used beyond the currently benefited fields of various sciences to benefit retail, manufacturing, consumerism and financial services. But how much data is enough data? The beauty of big data analysis is that it compensates for standard deviation and outliers by removing irregularities due to its mass.
The idea of skipping the methodical mathematics to create functions usable in the real world by modeling data and isolating trends is the epitome of the growing big data analysis field. By utilizing the collection of data, analyzing its results, correlating to find variable dependencies and verifying continuously, global statistics, industrial competitiveness, social trends and many other fields can be statistically predicted and optimized with increasingly greater accuracy. There is no doubt that as the methods of data capturing and analysis increase, big data will become more instrumental to businesses. As such it is important these methods continue to be perfected, simplified and applied to each possible segment of data that could be analyzed for increased understanding. See here where IBM, a leader in the field of big data analytics, introduces the usefulness of big data as an asset to the world in business and other areas.
Sources:
Duffy, J. (2012). The Big Problem With Big Data. PC Magazine, 1.
Gudivada, V. N., Baeza-Yates, R., & Raghavan, V. V. (2015). Big Data: Promises and Problems. Computer, 48(3), 20-23.
Hal R. Varian. Big Data: New Tricks for Econometrics. The Journal of Economic Perspectives. Vol. 28, No. 2 (Spring 2014), pp. 3-27
Jagadish H, Gehrke J, Shahabi C, et al. Big Data and Its Technical Challenges. Communications Of The ACM [serial online]. July 2014;57(7):86-94. Available from: Computers & Applied Sciences Complete, Ipswich, MA. Accessed March 31, 2015
Spiess, J., T’Joens, Y., Dragnea, R., Spencer, P., & Philippart, L. (2014). Using Big Data to Improve Customer Experience and Business Performance. Bell Labs Technical Journal, 18(4), 3-17.
~
Teaching with Computers
Guest Author: William Payne
One of the events that my school hosts every year is an ice cream social, where we welcome new students to the math department, and try and convince those who are unsure to join our ranks. I look forward to it every year, but this year was particularly important to me. It was spring of my senior year. I had pretty much survived both the Augustana Mathematics major and the Teaching Mathematics major. At this event, I had occasion to talk to the new computer science professor, a man with whom I’ve had very little dealings, talk a little bit about his philosophy of mathematics education. He argued that computer science was becoming the “new math,” since the kinds of things people needed to do with mathematics in real-world jobs involved being able to strategize technology to solve and deal with the astronomical numbers that often occur. Being a teacher-hopeful, I thought this was particularly interesting, and this professor really planted a seed.
Fast forward a few weeks, and I was looking for a mathematics video to expand my mathematical horizons. And lo and behold- one of the first ones I stumbled upon was a TED talk about teaching mathematics in schools through computers, done by none other than Conrad Wolfram. He frames his talk around a very simple question- why are we teaching mathematics? He argues that modern math educators have lost sight of this question and are just teaching calculations, and so students are getting disconnected from, even though the modern world needs it more than ever. He also says that there are three real uses for mathematics- modern day technical jobs, every day living, and logic.
He also believes that math should happen in three steps- posing the right question (more problems here), turn it into math problem, compute, and translate it back to real world. He believes that in the education field, there is too much focus on step three, and not enough on step one. He also argues that programming is a really important way of teaching math, and a student can really show deep understanding of something if they can program a computer to do it. He believes making this paradigm shift will benefit practical and conceptual at the same time, which is rare to find.
 Going into the educational field, I was enthralled by what he had to say, and I wanted to know more about what research says about the math field. For instance, the Wright article argues that a huge hole in the modern United States economy is the lack of familiarity with computer programming languages. Like Wolfram, the Computer Science Teachers Association say that computer programming will link to most other fields, will teach students how to really translate and deal with a problem, will increase engagement in a variety of subjects.
Going into the educational field, I was enthralled by what he had to say, and I wanted to know more about what research says about the math field. For instance, the Wright article argues that a huge hole in the modern United States economy is the lack of familiarity with computer programming languages. Like Wolfram, the Computer Science Teachers Association say that computer programming will link to most other fields, will teach students how to really translate and deal with a problem, will increase engagement in a variety of subjects.
The Feurzeig piece also argues that mathematics is often too abstract, and too removed from the ideas that people will need in real-world jobs. They give example of a programming language they developed, called LOGO, which was used in math education for younger students, which seemed to really engage them from a young age. It got them all using the same terminology, and being a specifically designed language, allowed for a less formal syntax than a different programming language might.
Looking forward, the idea that computer programming might find its way into the math curriculum excites me. There does seem to be a weakness coming from students with translating a problem into something that can be solved. However, all that being said, I think math educators have to be careful as to not allow the use of programming become as disconnected as math can often seem today. Mathematics is beautiful, and students should be taught to appreciate its beauty. I truly believe that integrating technology and programming into the math curriculum will lead to an increase in understanding, and I look forward to seeing how things change over the years.
Sources:
Feurzeig, Wallace, Seymour A. Papert, and Bob Lawler. “Programming-languages as a Conceptual
Framework for Teaching Mathematics.”Interactive Learning Environments 19.5 (2011): 487-501. Web. 12 Apr. 2015.
Wright, Geoffrey A., Peter Rich, and Keith R. Leatham. “How Programming Fits With
Technology Education Curriculum.” Technology & Engineering Teacher 71.7 (2012): 3-9. Academic Search Complete. Web. 12 Apr. 2015.
Image source: http://thenextweb.com/entrepreneur/2014/04/16/digital-disruption-changing-business-technology-isnt-answer/