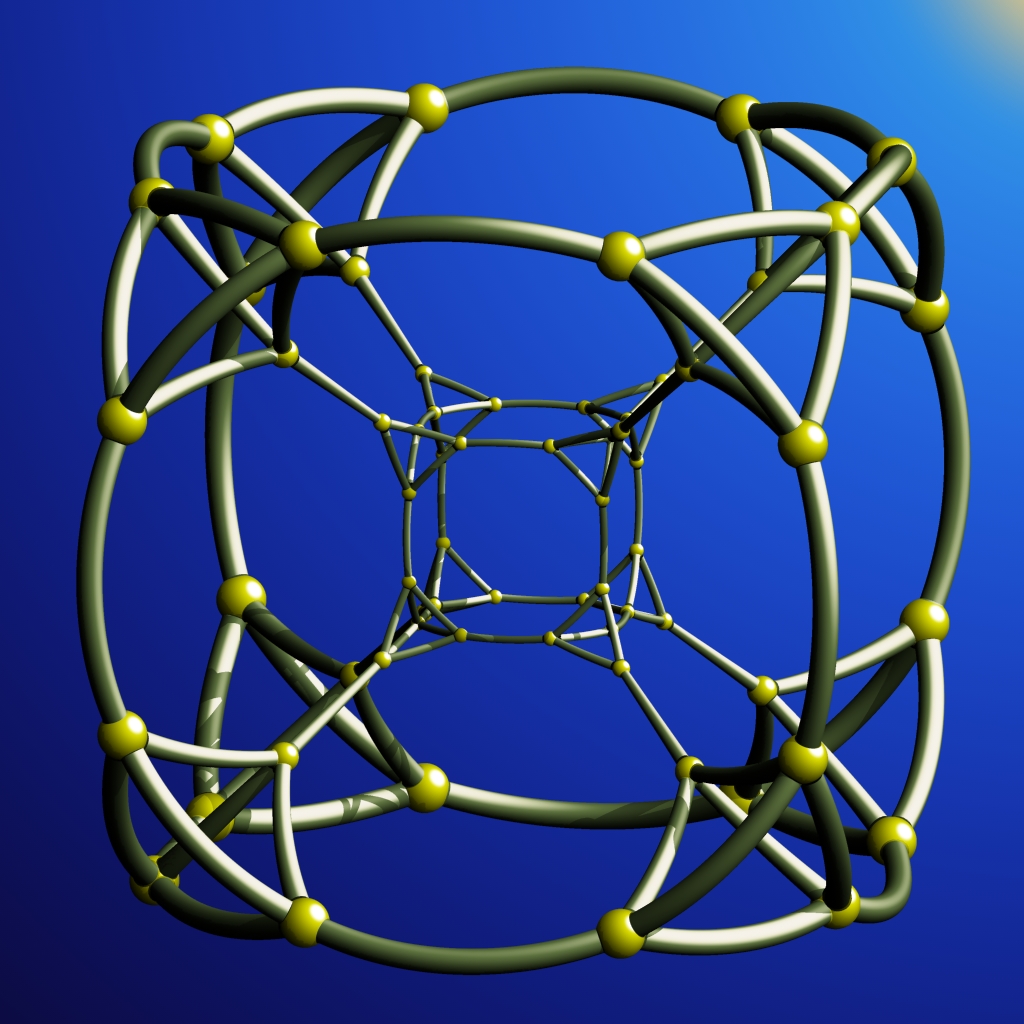
---

This is a truncated 4-dimensional cube. You can take an ordinary 3-dimensional cube, cut off its corners, and get a uniform polyhedron with $2 \times 3 = 6$ octagonal faces and $2^3 = 8$ triangular faces. It’s called the truncated cube. Similarly, you can take a 4-dimensional cube, cut off its corners, and get a 4d uniform polytope with $2 \times 4 = 8$ truncated cubes as facets and $2^4 = 16$ tetrahedral facets! It’s called the truncated 4-cube.

This particular truncated 4-cube was drawn in a curved style by Jos Leys. You can see more of his 4d polytopes here:

• Jos Leys, 4d Polychora.

A polychoron is just another name for a 4-dimensional polytope. The truncated 4-cube is also called the truncated tesseract, and you can learn more about it here:

• Truncated tesseract, Wikipedia.

The Coxeter diagram of the truncated 4d cube is

 

where the black dots are often drawn as dots with rings around them, and the white ones are often drawn as dots without rings. The unmarked diagram

 

describes the symmetry group of the 4-cube, including both rotations and reflections. This group, called a Coxeter group, has four generators $s_1, \dots, s_4$ obeying relations that are encoded in the diagram:

$$ (s_1 s_2)^4 = 1 $$
$$ (s_2 s_3)^3 = 1 $$
$$ (s_3 s_4)^3 = 1 $$

together with relations

$$s_i^2 = 1$$

and

$$ s_i s_j = s_j s_i \; \textrm{ if } \; |i – j| > 1 $$

Marking the Coxeter diagram lets us describe many uniform polytopes with the same symmetry group as the 4-cube. You can think of the 4 dots as corresponding to the vertices, edges, 2d faces and 3d facets of the cube. Blackening the vertex and edge dots:

 

is a way to indicate that the truncated 4-cube has a vertex for each vertex-edge flag: that is, each pair consisting of a vertex and an edge of the 4-cube, where the vertex lies on the edge.

All this generalizes from 4 dimensions to higher (or lower) dimensions. The truncated $n$-cube has $2n$ truncated $(n-1)$-cubes and $2^n$ $(n-1)$-simplices as faces, and it is described by a Coxeter diagram just like the one above, but with $n$ dots. For example, the truncated 5-cube has this diagram:

 

---

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!