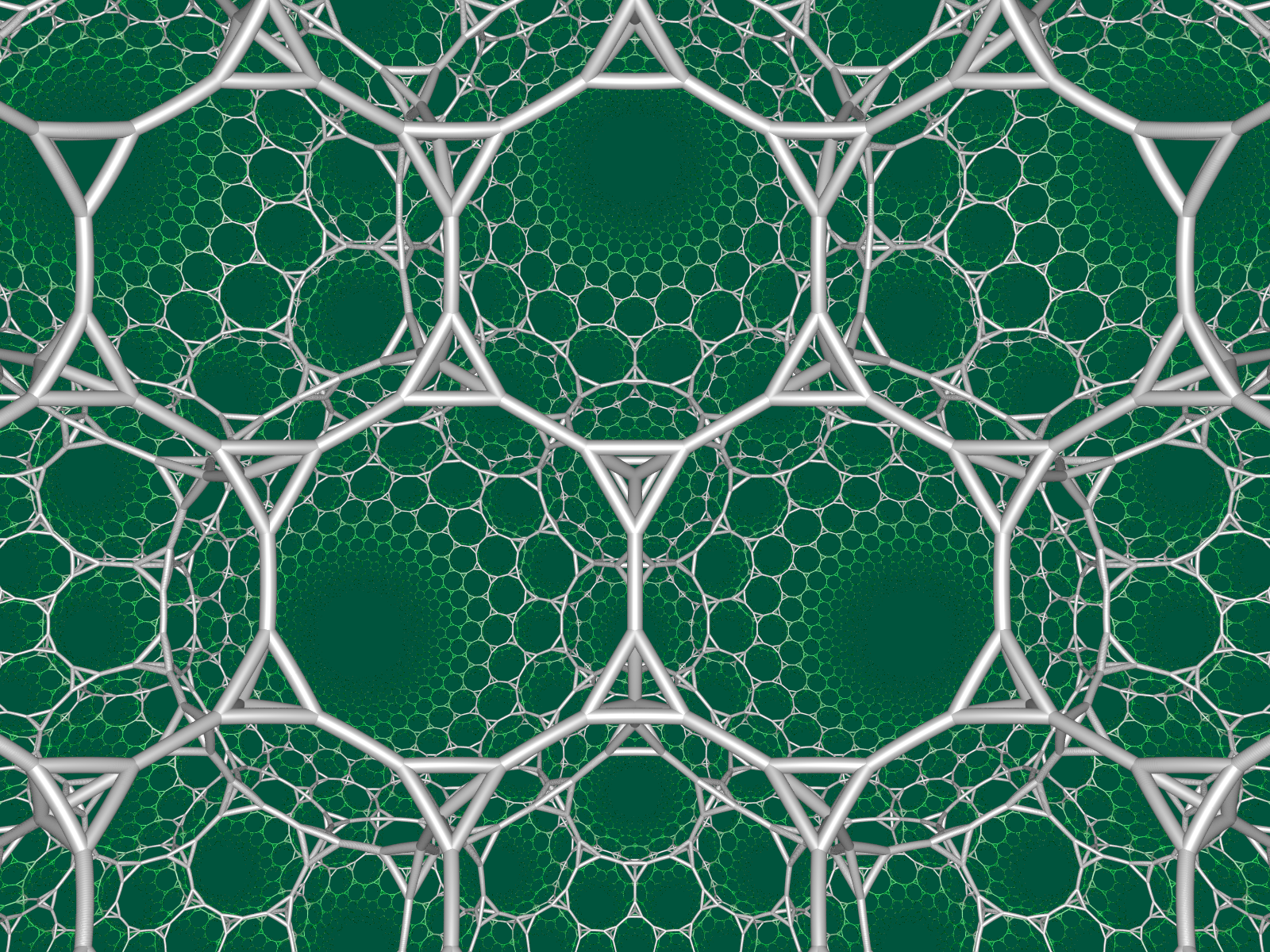
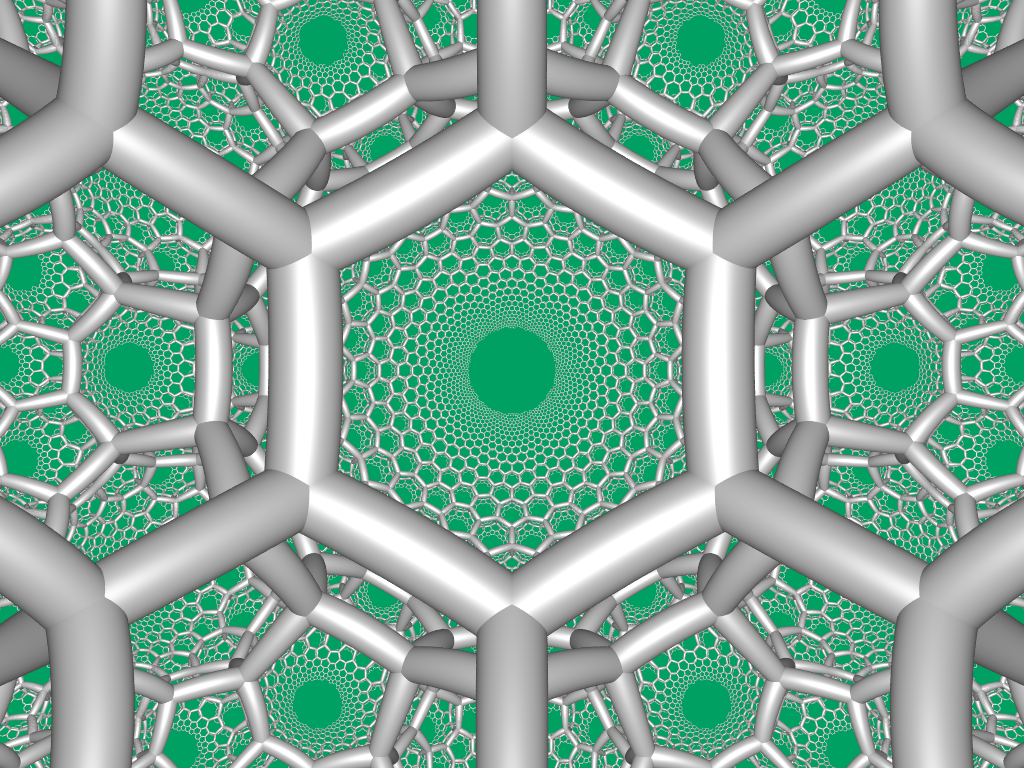
This is an image of the truncated {6,3,3} honeycomb, created by Roice Nelson. This honeycomb lives in a curved 3-dimensional space called hyperbolic space.

To understand the truncated {6,3,3} honeycomb, we need to start with the {6,3,3} honeycomb:

The {6,3,3} honeycomb is also called the hexagonal tiling honeycomb, because it contains sheets of hexagons tiling flat Euclidean planes embedded in hyperbolic space.

The notation {6,3,3} is an example of a Schläfli symbol. The Schläfli symbol is defined in a recursive way. The symbol for the hexagon is {6}. The symbol for the hexagonal tiling of the plane is {6,3} because 3 hexagons meet at each vertex. Finally, the hexagonal tiling honeycomb has symbol {6,3,3} because 3 hexagonal tilings meet at each edge.

Just as the {6,3} inside {6,3,3} describes the hexagonal tilings inside the {6,3,3} honeycomb, the {3,3} describes the vertex figure of this honeycomb: that is, the way the edges meet at each vertex. {3,3} is the Schläfli symbol for the regular tetrahedron, and if you look at the picture above you can can see that each vertex has 4 edges coming out, just like the edges going from the center of a tetrahedron to its corners.

To obtain the truncated {6,3,3} honeycomb, we replace each vertex of {6,3,3} honeycomb by a tetrahedron. We can think of this process as chopping off the vertices of the {6,3,3} tiling, or truncating it.

The the {6,3,3} honeycomb has this Coxeter diagram:

 

while the Coxeter diagram of the truncated {6,3,3} honeycomb is this:

 

The extra black dot here indicates that each vertex in the truncated {6,3,3} honeycomb corresponds to a vertex-edge flag in the {6,3,3} honeycomb: that is, a pair consisting of a vertex and an edge incident to that vertex.

Both these honeycombs have the same symmetry group, a discrete subgroup of the isometry group of hyperbolic space. This discrete group has generators and relations summarized by the unmarked Coxeter diagram:

 

This diagram says there are four generators $s_1, \dots, s_4$ obeying relations encoded in the edges of the diagram:

$$ (s_1 s_2)^6 = 1 $$
$$ (s_2 s_3)^3 = 1 $$
$$ (s_3 s_4)^3 = 1 $$

together with relations

$$s_i^2 = 1$$

and

$$ s_i s_j = s_j s_i \; \textrm{ if } \; |i – j| > 1 $$

Marking the Coxeter diagram in different ways lets us describe many honeycombs with the same symmetry group as the hexagonal tiling honeycomb—in fact, $2^4 – 1 = 15$ of them, since there are 4 dots in the Coxeter diagram. You can see some of these here:

• Hexagonal tiling honeycomb, Wikipedia.

For more on the hexagonal tiling honeycomb, see:

• {6,3,3} honeycomb, Visual Insight.

• {6,3,3} honeycomb in upper half space, Visual Insight.

Roice Nelson, the creator of both images on this page, is a software developer with a passion for exploring mathematics through visualization:

• Roice.

The image of the truncated {6,3,3} honeycomb was created by Roice Nelson and put on on Wikicommons under a Creative Commons Attribution-Share Alike 3.0 Unported license. The image of the {6,3,3} honeycomb was created by him and put on Wikicommons under the same type of license.

---

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!