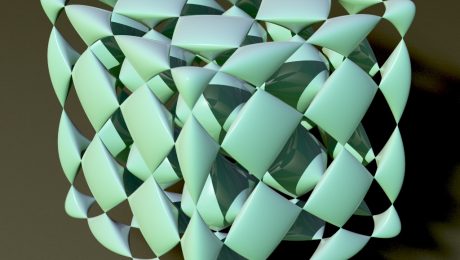
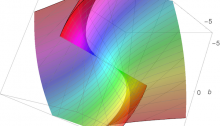
Chmutov Octic
An octic surface is one defined by a polynomial equation of degree 8. This image by Abdelaziz Nait Merzouk shows an octic discovered by Chmutov with 154 real ordinary double points or nodes: that is, points where it looks like the origin of the cone in 3-dimensional space defined by x2+y2=z2.