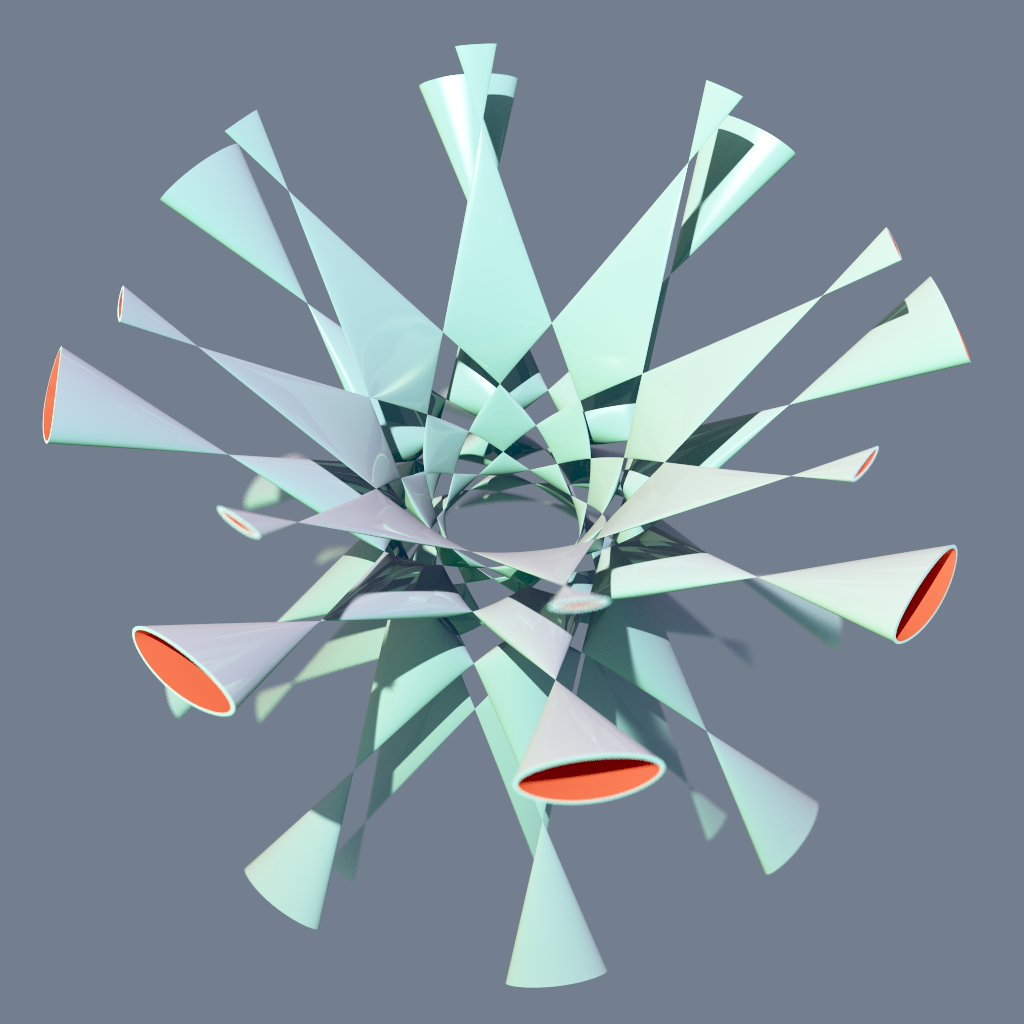
An octic surface is one defined by a polynomial equation of degree 8. The Endrass octic, drawn above by Abdelaziz Nait Merzouk, is currently the octic surface with the largest known number of ordinary double points: that is, points where it looks like the origin of the cone in 3-dimensional space defined by

$$ x^2 + y^2 = z^2 .$$

It has 168 ordinary double points, while the best known upper bound for a octic surface that’s smooth except for such singularities is 174.

The Endrass octic was discovered in 1995 by Stephan Endrass while he was writing his Ph.D. thesis under the direction of Wolf Barth. Barth was the discoverer of two other surfaces with the most ordinary double points for their degree: the Barth sextic and Barth decic, featured on previous posts here.

The Endrass octic actually comes in two versions. The version shown above, which we may call \(X^+\), is defined by this homogeneous polynomial equation of degree 8 in four variables \(w,x,y,z\):

$$ \begin{array}{c}
64 (x^2 – w^2) (y^2 – w^2) ((x+y)^2 – 2w^2) ((x-y)^2 – 2w^2) \; = \; \\
\left( -4(1 + \sqrt{2})((x^2 + y^2)^2 \; + \; \left(8(2 + \sqrt{2})z^2 + 2(2 + 7 \sqrt{2})w^2\right)(x^2 + y^2) \right. \\ \left. – \; 16z^4 \; + \; 8(1 – 2 \sqrt{2})z^2w^2 \; – \; (1 + 12 \sqrt{2})w^4 \right)^2
\end{array} $$

Endrass found this complicated equation with the help of a computer algebra system (Maple).

This equation determines a subset \(S \subset \mathbb{C}^4\) with complex dimension 2. Note that if \((w, x, y, z \in \mathbb{C}^4 \) is a solution, so is any multiple \((cw, cx, cy, cz) \). We may thus projectivize \(S\), treating any solution as ‘the same’ as any multiple of that solution. The result is an algebraic variety \(X^+\) in the complex projective space \(\mathbb{C}\mathrm{P}^3\). This variety has complex dimension 2, so it is called a complex surface. To obtain an ordinary real 2-dimensional surface we may take its intersection with a copy of \(\mathbb{R}\mathrm{P}^3\) in \(\mathbb{C}\mathrm{P}^3\).

Sitting inside \(\mathbb{R}\mathrm{P}^3\) we in turn have many copies of ordinary 3-dimensional space, \(\mathbb{R}^3\). The picture above shows the portion of the Endrass octic living in one of these copies. Concretely, this consists of real solutions of the above equation where \(w = 1\).

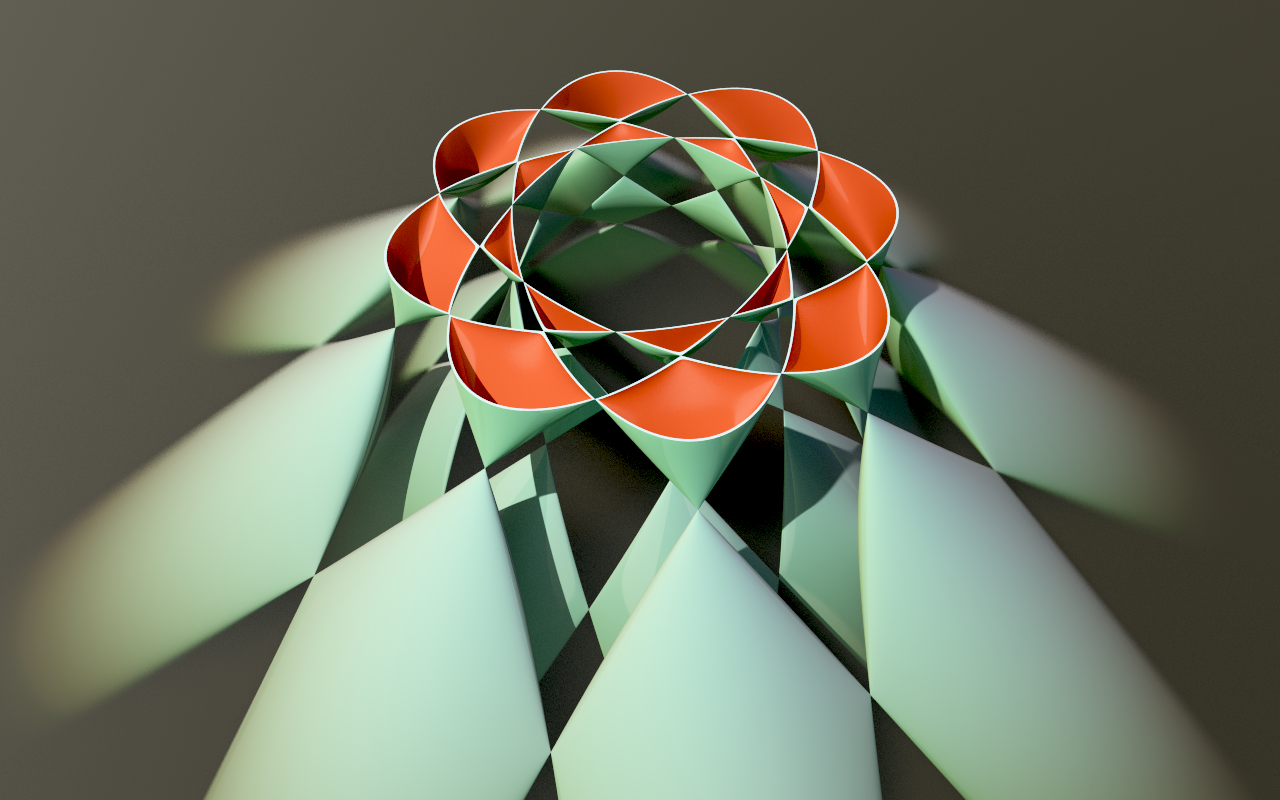
Here is a picture of the Endrass octic \(X^+\) cut by the ‘plane at infinity’:

The plane at infinity is the complement of \(\mathbb{R}^3\) inside \(\mathbb{R}\mathrm{P}^3\). This is obtained by projectivizing the hyperplane \(w = 0\). As you can see, some of the double points lie in the plane at infinity, rather than inside \(\mathbb{R}^3\).

Puzzle 1. How many double points of \(X^+\) lie in the plane at infinity?

Puzzle 2. The number 168 is famous as the order of the a second smallest nonabelian finite simple group, \(\mathrm{PSL}(2,\mathbb{F}_7)\). Is there any connection between this group and the Endrass octic \(X^+\)?

There is a second Endrass octic that also has 168 ordinary double points. We may call it \(X^-\), because it is obtained by taking the equation for \(X^+\) and replacing \(\sqrt{2}\) by \(-\sqrt{2}\) throughout. So, the equation for \(X^-\) is this:

$$ \begin{array}{c}
64 (x^2 – w^2) (y^2 – w^2) ((x+y)^2 – 2w^2) ((x-y)^2 – 2w^2) \; = \; \\
\left(-4(1 – \sqrt{2})((x^2 + y^2)^2 \; + \; \left(8(2 – \sqrt{2})z^2 + 2(2 + 7 \sqrt{2})w^2\right)(x^2 + y^2) \right. \\ \left. – \; 16z^4 \; + \; 8(1 + 2 \sqrt{2})z^2w^2 \; – \; (1 – 12 \sqrt{2})w^4 \right)^2
\end{array} $$

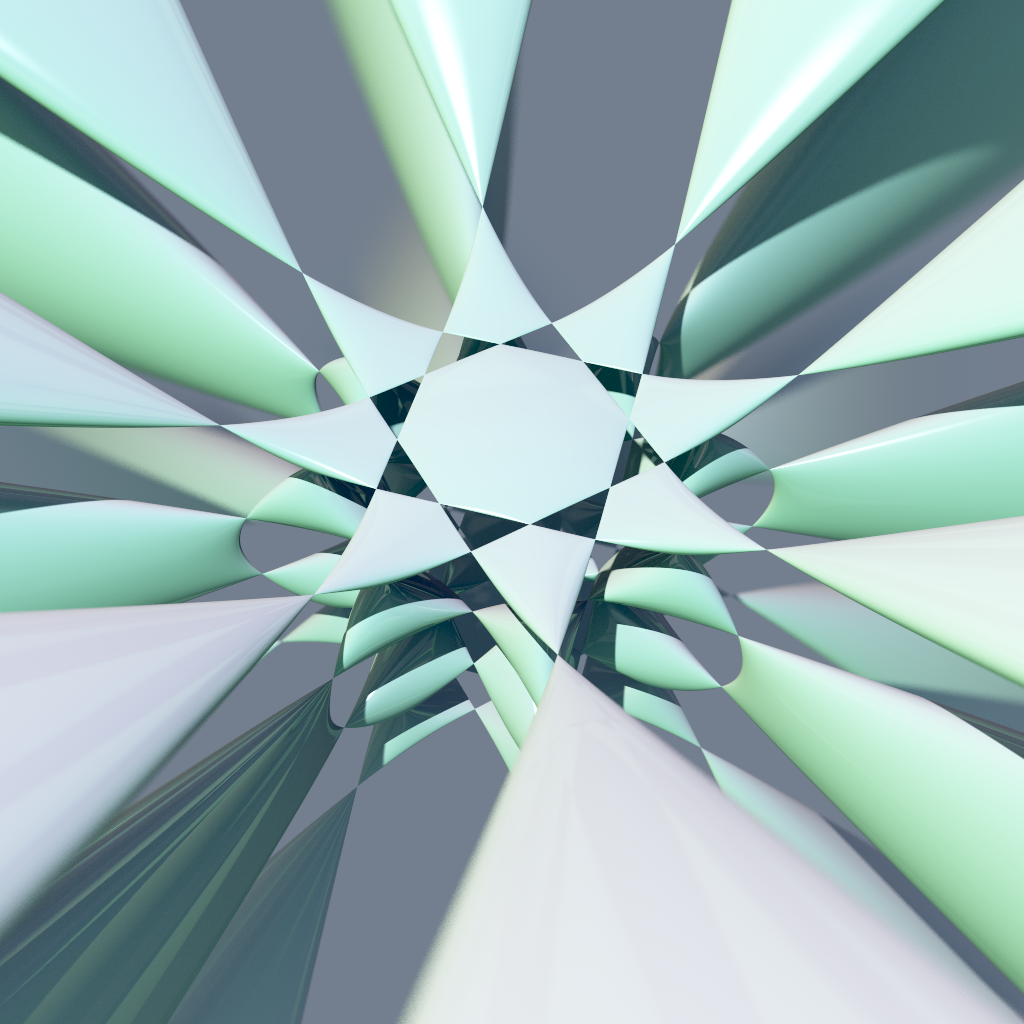
While all 168 double points of \(X^+\) are real, 24 are complex for \(X^-\). Here is a picture of \(X^-\) drawn by Abdelaziz Nait Merzouk:

Again, this shows real solutions of the equation where \(w = 1\).

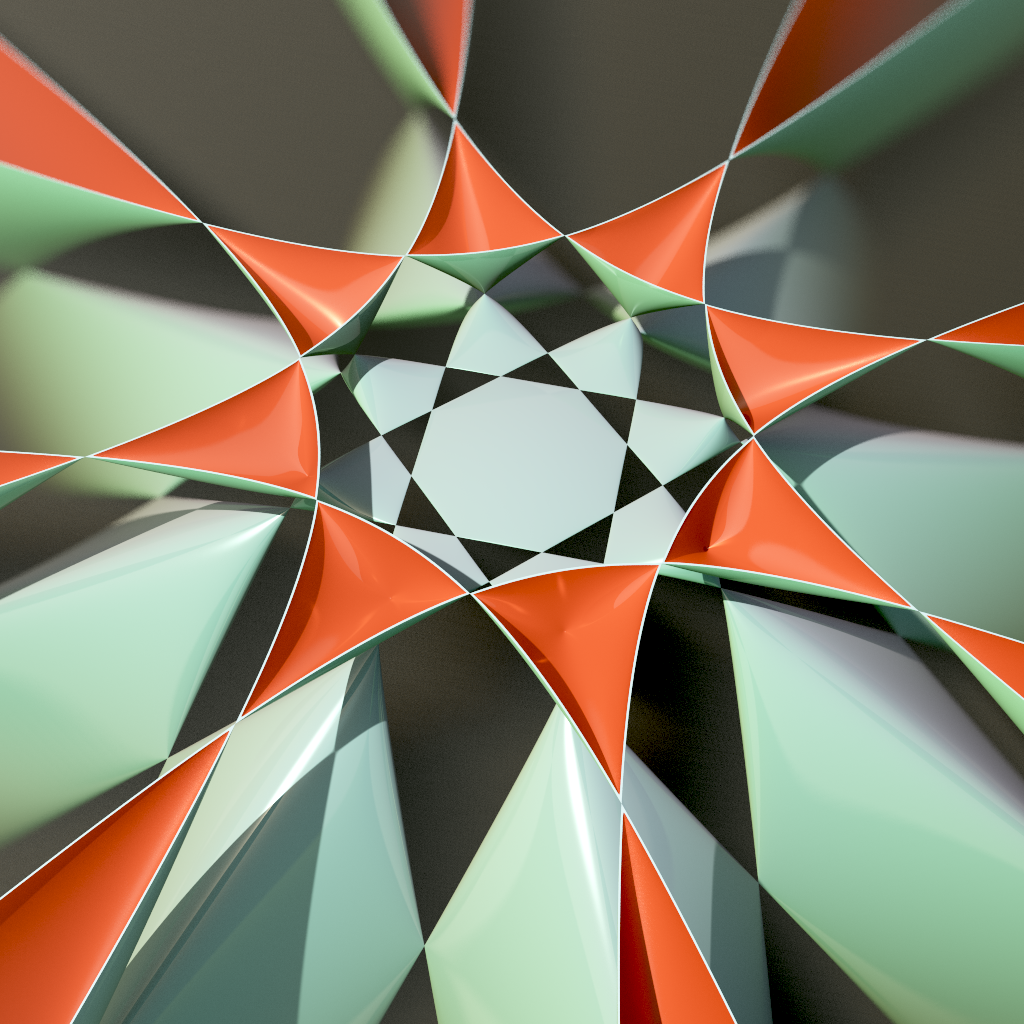
Here is a view of \(X^-\) that has been rotated by 90° in the \(zw\) plane and cut by the image of the plane at infinity:

The following animation shows a version of \(X^-\) rotating in the \(zw\) plane, so that points at infinity are brought into view. It also displays more of the overall structure of \(X^-\):

Endrass discovered his octics inside a five-dimensional family of octics. All the octics in this family have at least 112 ordinary double points and are invariant under the group \(\mathrm{D}_8 \times \mathbb{Z}/2\). They are eightfold covers of quartic surfaces.

For more details see:

• Stephan Endrass, Flächen mit vielen Doppelpunkten, DMV-Mitteilungen 4 (1995), 17–20.

• Stephan Endrass, A projective surface of degree eight with 168 nodes, J. Algebraic Geom. 6 (1997), 325–334.

For more pictures, see:

• Stephan Endrass, Octics with 168 nodes.

In 1984, Miyaoka proved that an octic surface in $\mathbb{C}\mathrm{P}^3$ with only rational double points can have at most 174 such points. This is currently still the best known upper bound:

• Y. Miyaoka, The maximal number of quotient singularities on surfaces with given numerical invariants, Math. Ann. 268 (1984), 159–171.

---

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!