Kummer Quartic Surface – Abdelaziz Nait Merzouk

A quartic surface is one defined by a polynomial equation of degree 4. A nodal surface is one whose only singularities are ordinary double points: that is, points where it looks like the origin of the cone in 3-dimensional space defined by

$$ x^2 + y^2 = z^2 .$$

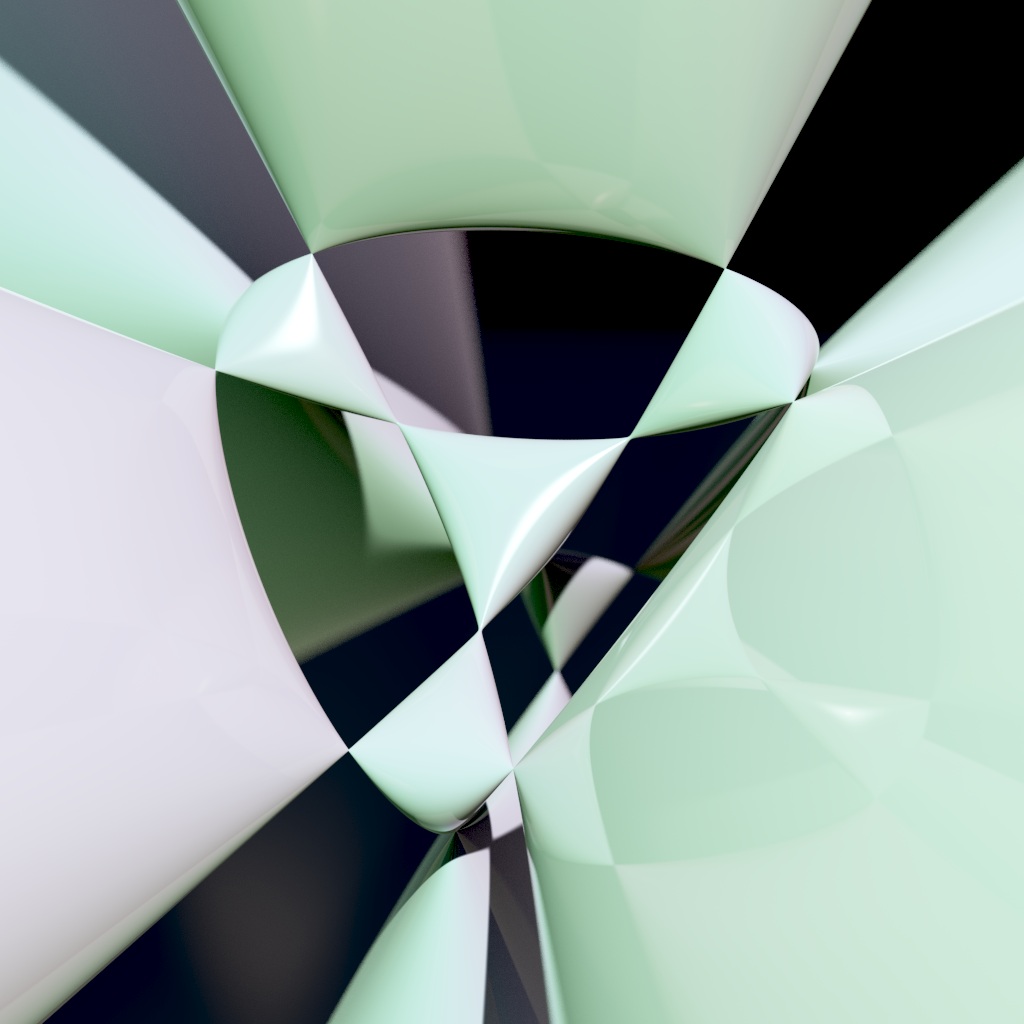
The Kummer surfaces are the quartic nodal surfaces with the largest possible number of ordinary double points, namely 16. In the above picture, Abdelaziz Nait Merzouk has drawn the real points of a Kummer surface.

There are many different Kummer surfaces, but they all have the same topology and they can all be constructed in the same way. Start with a smooth complex curve of genus 2, say \(C\):

Genus Two Curve – Oleg Alexandrov

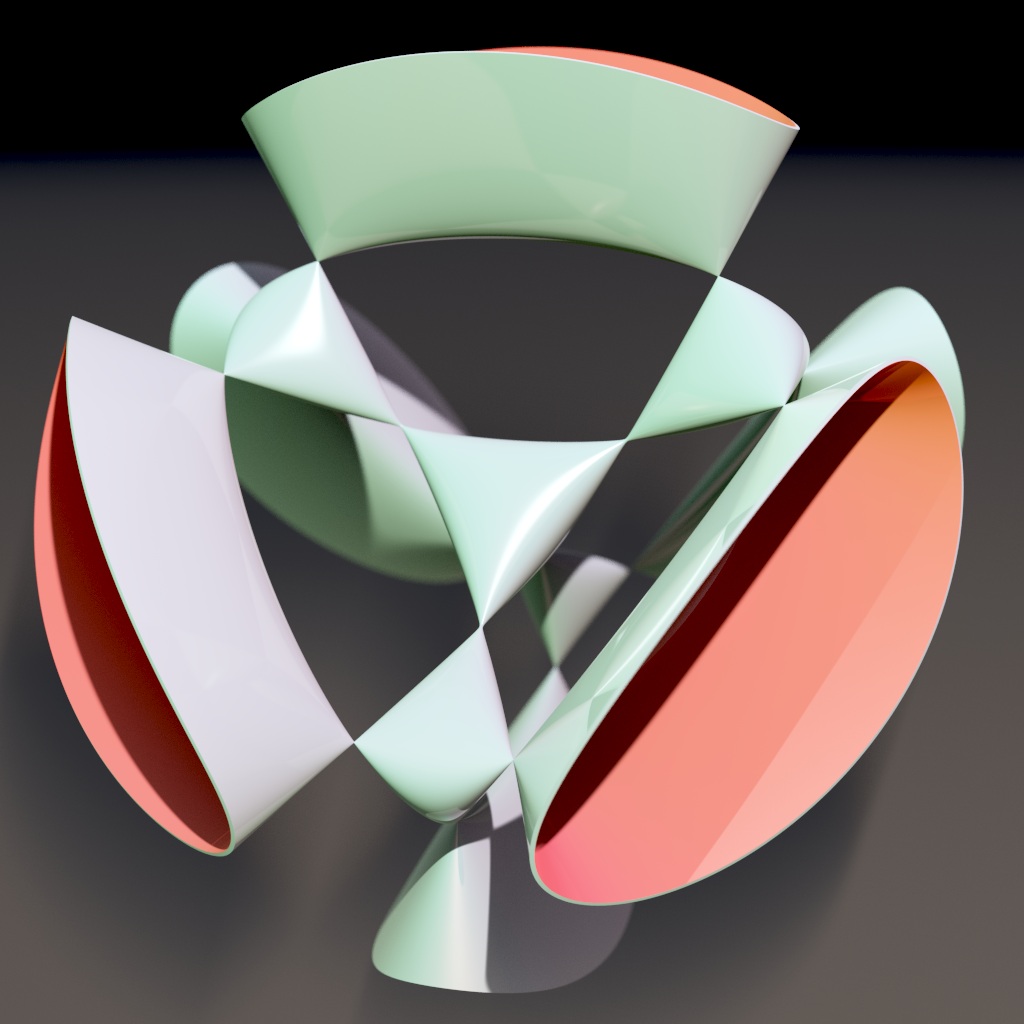
Then, form its Jacobian variety \(J(C)\). This is the space of isomorphism classes of holomorphic line bundles over \(C\) with vanishing first Chern class. As a topological space, this Jacobian is always product of 4 copies of a circle, but it is equipped with the structure of a complex surface in a way that depends on \(C\). It is an abelian group, thanks to our ability to tensor line bundles, so it has an automorphism \(x \mapsto -x\) called the Kummer involution. The quotient of the Jacobian by this involution is a Kummer surface. The ordinary double points come from the points in \(J(C)\) with \(x = -x\); there are \(16 = 2^4\) of these in a product of 4 copies of the circle group.

Here is a ‘cut’ view of the same Kummer surface. Again, only the real points are shown:

Cut Kummer’s Quartic Surface – Abdelaziz Nait Merzouk

For an introduction to Kummer surfaces, see:

• Dylan Wilson, Introducing K3 surfaces: Kummer surfaces, 2014.

Explicit equations for the Kummer surfaces can be found here:

• Kummer surface, Wolfram MathWorld.

Abdelaziz Nait Merzouk created these pictures of a Kummer surface and made them available on Google+ under a Creative Commons Attribution-ShareAlike 3.0 Unported license. Oleg Alexandrov made the picture of a complex curve of genus two and put it in the public domain on Wikicommons.

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!