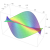
This is the {6,3,6} honeycomb, drawn by Roice Nelson.

A 3-dimensional honeycomb is a way of filling 3d space with polyhedra or infinite sheets of polygons. Besides honeycombs in 3d Euclidean space, we can also have honeycombs in 3d hyperbolic space, a non-Euclidean geometry with constant negative curvature. The {6,3,6} honeycomb lives in hyperbolic space.

The {6,3,6} honeycomb is also called the order 6 hexagonal tiling honeycomb. The reason is that it’s built from sheets of regular hexagons, with 6 sheets meeting along each edge of the honeycomb. It is worth comparing the {6,3,3}, {6,3,4} and {6,3,5} honeycombs, which have 3, 4 or 5 sheets of hexagons meeting along each edge. We’ve met these in previous posts:

• {6,3,3} honeycomb, Visual Insight.

• {6,3,4} honeycomb, Visual Insight.

• {6,3,5} honeycomb, Visual Insight.

All these honeycombs are paracompact: instead of polyhedra, they contain infinite sheets tiled by polygons. But the {6,3,6} is the last of this series, and the most special.

The reason is that this honeycomb is self-dual. The tetrahedron is a simpler example of a self-dual shape. If we draw a vertex in the middle of each face of the tetrahedron, and draw an edge crossing each edge, we get a new shape with a face for each vertex of the tetrahedron… but this new shape is again a tetrahedron!

If we do a similar thing one dimension up for the {6,3,6} honeycomb, this amounts to creating a new honeycomb with:

• one vertex for each infinite sheet tiled with hexagons in the original honeycomb;
• one edge for each hexagon in the original honeycomb;
• one hexagon for each edge in the original honeycomb;
• one infinite sheet tiled with hexagons for each vertex in the original honeycomb.

But this new honeycomb turns out to be another {6,3,6} honeycomb!

This is hard to visualize (at least for me), but one thing it implies is this. Just as infinite sheet has infinitely many hexagons on it, each vertex has infinitely many edges going through it.

This self-duality comes from the symmetry of the so-called Schläfli symbol {6,3,6}: if you reverse it, you get the same thing! We can also see this symmetry in the Coxeter diagram of the {6,3,6} honeycomb:

 
For more on what this means, see the above Visual Insight articles on honeycombs. For more on this particular honeycomb and its close relatives, see:

• Order-6 hexagonal tiling honeycomb, Wikipedia.

Puzzle: Why is the {6,3,6} honeycomb the last of this series of hyperbolic honeycombs? What do you get if you try to built {6,3,7}?

I don’t know, but it’s related to this:

• You can build a tetrahedron where 3 triangles meet at each corner. For this reason, the Schl´fli symbol of the tetrahedron is {3,3}. You can build a hyperbolic honeycomb where the edges coming out of any vertex go out to the corners of a tetrahedron… and these edges form hexagons. This is the {6,3,3} honeycomb.

• You can build an octahedron where 4 triangles meet at each corner. Thus, the Schl´fli symbol of the octahedron is {3,4}. You can build a hyperbolic honeycomb where the edges coming out of any vertex go out to the corners of an octahedron… and these edges form hexagons. This is the {6,3,4} honeycomb.

• You can build an icosahedron where 5 triangles meet at each corner. Thus, the Schl´fli symbol of icosahedron is {3,5}. You can build a hyperbolic honeycomb where the edges coming out of any vertex go out to the corners of an icosahedron… and these edges form hexagons. This is the {6,3,5} honeycomb.

• You can build a tiling of a flat plane where 6 triangles meet at each corner. The Schl´fli symbol of this triangular tiling is {3,6}. You can build a hyperbolic honeycomb where the edges coming out of any vertex go out to the corners of a triangular tiling… and these edges form hexagons. This is the {6,3,6} honeycomb.

The last one is different from the rest, and when we reach {3,7} we get a triangular tiling of the hyperbolic plane. What does this imply about {6,3,7}?

Puzzle: The symmetry group of 3d hyperbolic space, not counting reflections, is $\mathrm{PSL}(2,\mathbb{C})$. Can you explicitly describe the subgroup that preserves the order-6 hexagonal tiling honeycomb?

Roice Nelson, the creator of this image, has a blog with lots of articles about geometry, and he makes plastic models of interesting geometrical objects using a 3d printer:

• Roice.

---

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!