Labs Septic – Abdelaziz Nait Merzouk

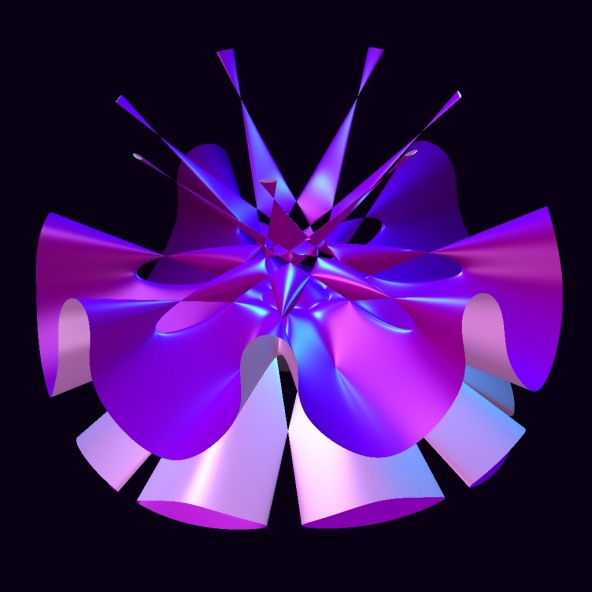
A septic surface is one defined by a polynomial equation of degree 7. The Labs septic, drawn above by Abdelaziz Nait Merzouk, is the septic surface with the maximum known number of ordinary double points: that is, points where it looks like the origin of the cone in 3-dimensional space defined by

$$ x^2 + y^2 = z^2 .$$

It has 99 ordinary double points.

The Labs septic was discovered by Oliver Labs while he was writing his Ph.D. thesis under the direction of Duco van Straten. The construction is explained here:

• Oliver Labs, A septic with 99 real nodes, Rend. Sem. Mat. Univ. Padova 116 (2006), 299–313.

In a nutshell, he studied a 7-parameter family of septic surfaces having the dihedral group \(\mathrm{D}_7\) as symmetries. Using the computer algebra program Singular, he found candidate surfaces having large numbers of ordinary double points over small finite fields. Then he examined these candidates over the complex numbers. The Labs septic actually has 100 ordinary double points over an algebraic extension of $\mathbb{F}_5.$ Of these double points, 99 survive over the complex numbers, and all of these are defined over the real numbers.

The explicit formula for the Labs septic is rather complicated, but it can be found in his paper. The current best upper bound on the maximum number of double points for a septic surface is 104. This was first proved by Varchenko:

• Alexander N. Varchenko, On the semicontinuity of the spectrum and an upper bound for the number of singular points of a projective hypersurface, J. Soviet Math. 27 (1983), 735–739.

Here is a picture of this surface drawn by Oliver Labs himself:

Labs Septic – Oliver Labs

This picture was made available with a Creative Commons Attribution-NonCommercial-ShareAlike 3.0 Unported license. Labs has also made available a mpg file containing a movie of his septic, which makes it easier to visualize. See also:

• Oliver Labs, Septics with many singularities.

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!