Origami Dodecahedra – Greg Egan

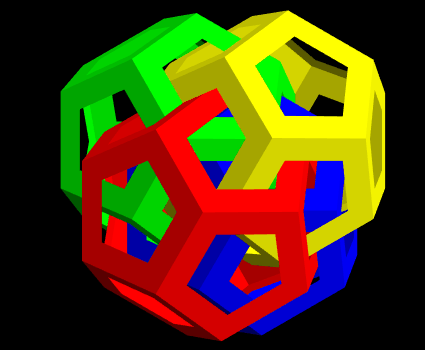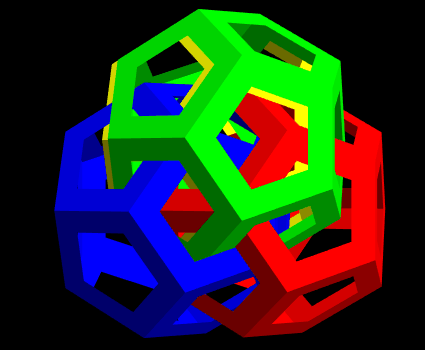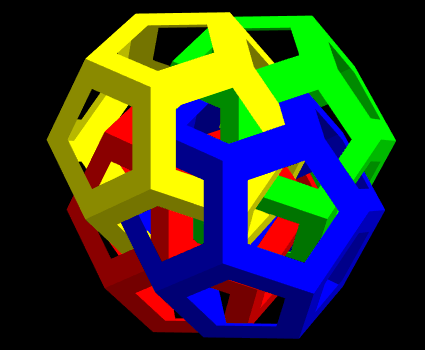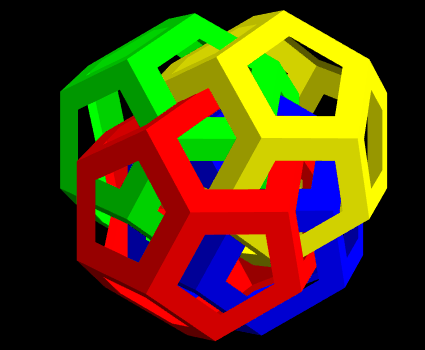
There is a nice photograph of some interlocking origami dodecahedra created by Dirk Eisner on the website Mathematical Origami. But it’s hard to be sure how many dodecahedra there are, since some could be hidden from view. This raises a puzzle: assuming the configuration is as symmetrical as possible, how many dodecahedra are there?

Greg Egan answered this with the spectacular image above! It shows four dodecahedra whose centers lie at the vertices of a regular tetrahedron.

However, Egan went further. He showed that this is the configuration of interlocking dodecahedra that makes the origami strips as wide as possible without intersecting. Without assuming the answer will be symmetrical, this requires a large computer search of a 9-dimensional space. One portion of this search required checking

$$ \binom{44}{10} = 2,481,256,778 $$

possible maxima!

However, the answer turns out to be highly symmetrical. Start with a regular dodecahedron. It has 20 vertices, and we can partition these into 5 sets of 4, each 4 being the vertices of a tetrahedron. Choose one of these tetrahedra, and rescale it by a factor of $1/\sqrt{5}$ while keeping its center fixed. We can take our dodecahedron and translate it in 4 different directions to obtain 4 dodecahedra whose centers lie at the vertices of this shrunken tetrahedron. This is what you see above.

In this solution, if the dodecahedra have edges of length 1, the distance between their centers is

$$ \sqrt{\frac{3 + \sqrt{5}}{5}} \approx 1.02333 $$

and the maximum possible strip width is

$$ \sqrt{\frac{1 – 1/\sqrt{5}}{8}} \approx 0.262866 $$

For more, visit:

• Greg Egan, Optimised origami.

While Egan needed a large computer search to solve the problem without symmetry assumptions, the problem becomes much easier if we assume symmetry from the start, and he also explains how to tackle this simpler variant.

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!