Togliatti Quintic – Abdelaziz Nait Merzouk

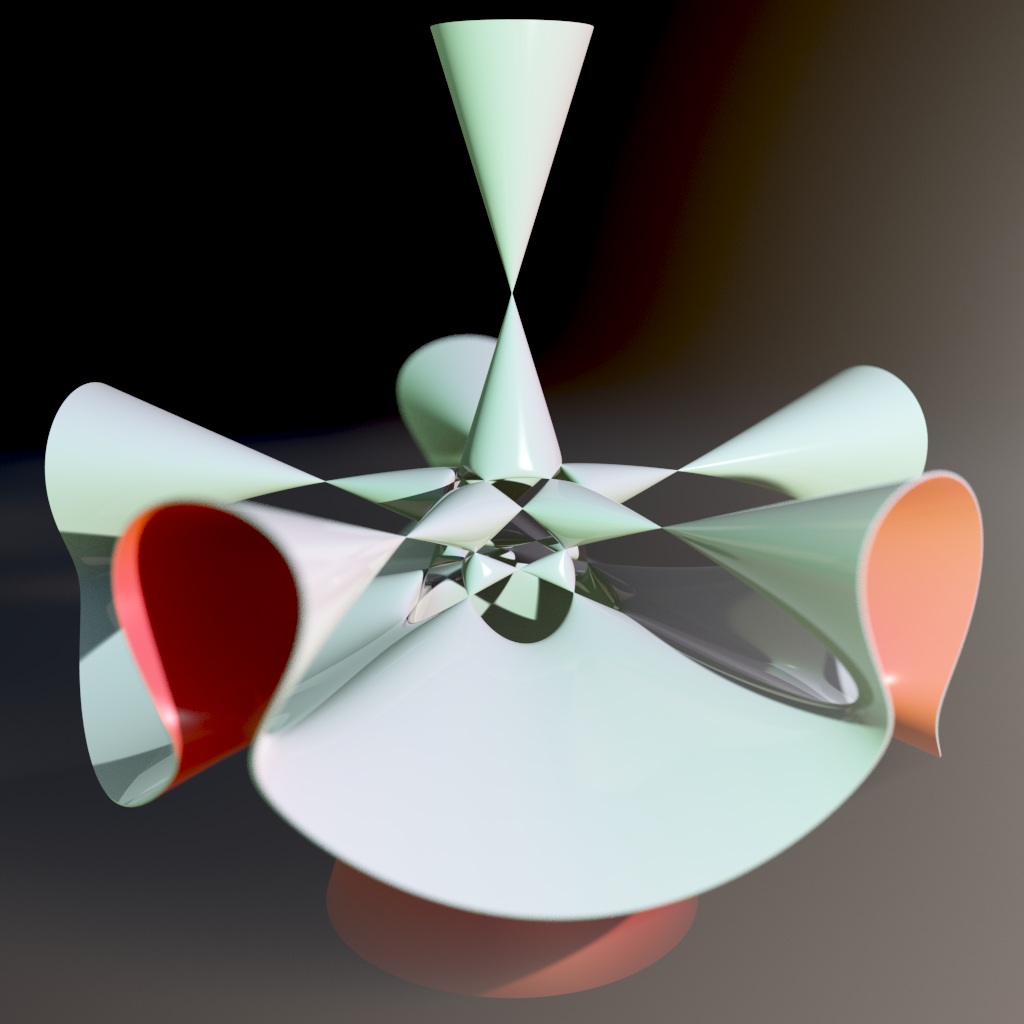
A quintic surface is one defined by a polynomial equation of degree 5. A nodal surface is one whose only singularities are ordinary double points: that is, points where it looks like the origin of the cone in 3-dimensional space defined by

$$ x^2 + y^2 = z^2 .$$

A Togliatti surface is a quintic nodal surface with the largest possible number of ordinary double points, namely 31. In the above picture, Abdelaziz Nait Merzouk has drawn the real points of a Togliatti surface.

This surface is described by a homogeneous quintic equation in four variables, say \(w,x,y,z\), which is then intersected with the hyperplane \(w = 1\). Here is a version rotated around the \(wz\) plane before intersecting with the hyperplane \(w = 1\):

Rotated Togliatti Quintic – Abdelaziz Nait Merzouk

This version is sometimes called the ‘dervish’, due to its resemblance to a whirling dervish.

The first example of a Togliatti quintic was constructed in 1940:

• Eugenio G. Togliatti, Una notevole superficie di 5° ordine con soli punti doppi isolati, Vierteljschr. Naturforsch. Ges. Zürich 85 (1940), 127–132.

In 1980, Beauville proved that 31 is the maximum possible number of nodes for a surface of this degree, showing this example to be optimal:

• Arnaud Beauville, Sur le nombre maximum de points doubles d’une surface dans \(\mathrm{P}^3\) (μ(5) = 31), Journées de Géometrie Algébrique d’Angers, Juillet 1979/Algebraic Geometry, Angers, 1979, Alphen aan den Rijn—Germantown, Md.: Sijthoff & Noordhoff, 1980, pp. 207–215.

Abdelaziz Nait Merzouk created these pictures of a Kummer surface and made them available on Google+ and made them available under a Creative Commons Attribution-ShareAlike 3.0 Unported license.

Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!