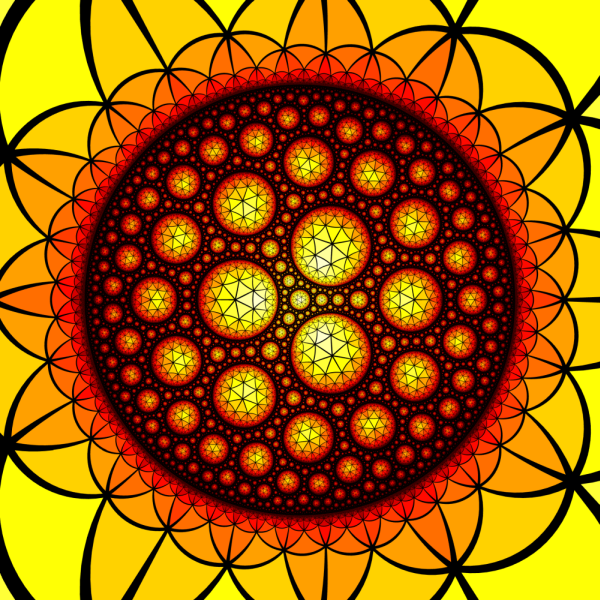
The {3,3,7} honeycomb is a honeycomb in 3d hyperbolic space. It is the dual of the {7,3,3} honeycomb shown last time:
The image above, drawn by Roice Nelson, shows the ‘boundary’ of the {3,3,7} honeycomb: that is, the set of points on the ‘plane at infinity’ of hyperbolic space that are limits of points in the {3,3,7} honeycomb.
Roice Nelson, the creator of this image, has a blog with lots of articles about geometry, and he makes plastic models of interesting geometrical objects using a 3d printer:
• Roice.
Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!