{5,3,5} Honeycomb – Jos Leys – www.josleys.com
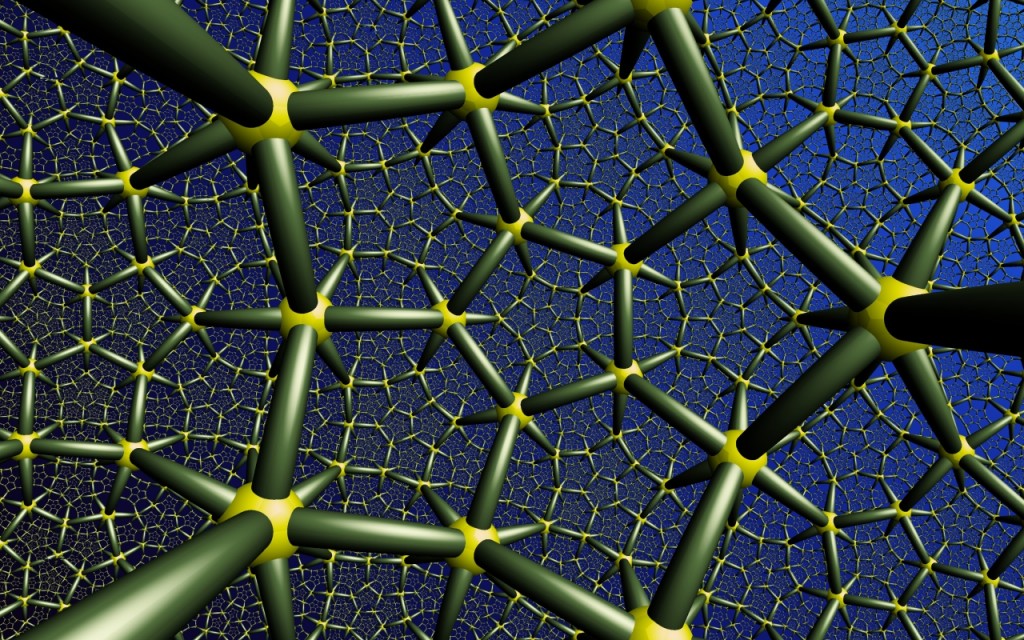
This is the {5,3,5} honeycomb, drawn by Jos Leys.
A 3-dimensional honeycomb is a way of filling 3d space with polyhedra. It’s the 3-dimensional analogue of a tiling of the plane. Besides honeycombs in Euclidean space, we can also have honeycombs in hyperbolic space, which is a 3-dimensional Riemannian manifold with constant negative curvature. The {5,3,5} honeycomb lives in hyperbolic space.
The {5,3,5} honeycomb is also called the order-5 dodecahedral honeycomb. The reason is that five dodecahedra meet at any edge of this honeycomb. This fact is recorded in the notation {5,3,5}, which is an example of a Schläfli symbol. The Schläfli symbol is defined in a recursive way. The symbol for the pentagon is {5}. The symbol for the regular dodecahedron is {5,3} because 3 pentagons meet at each vertex. Finally, the order-5 dodecahedral honeycomb has symbol {5,3,5} because 5 dodecahedra meet along each edge.
The {5,3,5} honeycomb is closely related to the Seifert–Weber space, which is a compact hyperbolic manifold. The Seifert–Weber space can be constructed by gluing each face of a dodecahedron to its opposite. There are three ways to do this gluing and get a compact 3-manifold. The opposite faces of the dodecahedron are misaligned by 1/10 of a turn, so to match them we must rotate them by 1/10, 3/10 or 5/10 of a turn. Rotating them by a 3/10 turn gives the Seifert–Weber space.
In the Seifert–Weber space, the edges of the original dodecahedron are glued to each other in groups of five. Thus, in this space, each edge is surrounded by five pentagonal faces, so the dihedral angle between these pentagons is $2 \pi / 5$.
This is also the dihedral angle of the dodecahedra in the {5,3,5} honeycomb, since 5 dodecahedra meet along each edge in this honeycomb. And indeed, the Seifert–Weber space is a quotient space of the {5,3,5} honeycomb! The {5,3,5} honeycomb is the universal cover of the Seifert–Weber space.
The {5,3,5} honeycomb is one of 15 regular honeycombs in hyperbolic space. For a complete list, with links to pictures, see:
• Tesselations of hyperbolic 3-space, Wikipedia.
Jos Leys has many nice pictures of hyperbolic honeycombs on his website:
• Jos Leys, Hyperbolic tesselations in 3d.
A nice feature of the {5,3,5} honeycomb is that it is self-dual: if we put a dot in the center of each dodecahedron and connect each dot to its neighbors, we get the vertices and edges of another {5,3,5} honeycomb! This property is visible in the palindromic nature of the Schläfli symbol {5,3,5}. The cubic honeycomb in Euclidean space is also self-dual, and it has Schläfli symbol {4,3,4}.
Coxeter diagrams are a notation similar to but more versatile than Schläfli symbols. The Coxeter diagram of the {5,3,5} honeycomb is
where the black dots are often drawn as dots with rings around them, and the white ones are often drawn as dots without rings.
The symmetry group of the {5,3,5} honeycomb is a discrete subgroup of the symmetry group of hyperbolic space. This discrete group has generators and relations summarized by the Coxeter diagram
Puzzle: The group $\mathrm{SL}(2,\mathbb{C})$ double covers the identity component of the Lorentz group $\mathrm{SO}^+(3,1)$, which is the symmetry group of hyperbolic space, not including reflections. What is an explicit description of the subgroup of $\mathrm{SL}(2,\mathbb{C})$ that double covers the symmetry group of {5,3,5}, excluding reflections?
For more on how this works, try this related example:
• Truncated hypercube, Visual Insight.
Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!
