Tübingen Tiling – Greg Egan
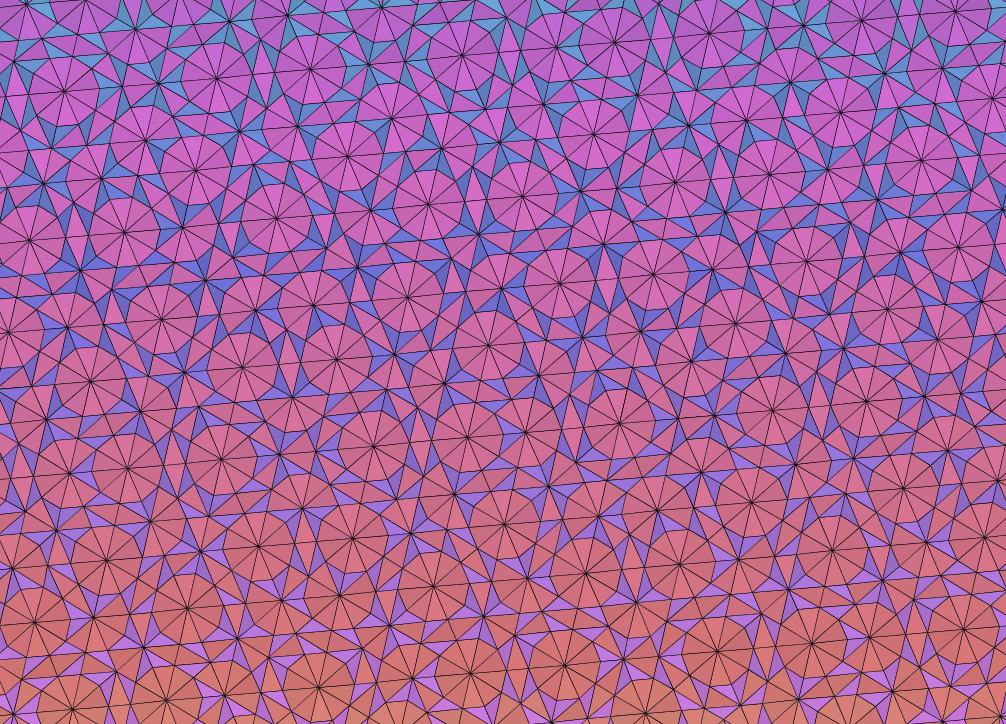
A systematic way to generate quasiperiodic tilings of the plane is to take a lattice in higher dimensions and slice it at a funny angle. Greg Egan’s Tübingen applet generates quasiperiodic tilings by projecting selected triangles from an $n$-dimensional lattice called the $\mathrm{A}_n$ lattice onto a plane. This particular picture comes from the $\mathrm{A}_4$ lattice. The applet produces moving pictures that are much more beautiful than this still image, so please check it out!
The $\mathrm{A}_n$ lattice lives in $n$ dimensions, but it’s easiest to describe it in one more dimension, as the set of all $(n+1)$-tuples of integers $(x_1,…,x_{n+1})$ such that
$$ x_1 + \cdots + x_{n+1} = 0. $$
It’s a fun exercise to show that $\mathrm{A}_2$ is a 2-dimensional hexagonal lattice, the sort of lattice you use to pack pennies as densely as possible. Similarly, $\mathrm{A}_3$ gives a standard way of packing grapefruit, which is in fact the densest lattice packing of spheres in 3 dimensions. If you were stacking layers of 4-dimensional grapefruit you could use the $\mathrm{A}_4$ lattice, though that would not be the densest possible packing.
Let me rapidly sketch how we get from the $\mathrm{A}_4$ lattice to the beautiful tiling shown here.
Each point $x$ in the $\mathrm{A}_4$ lattice is surrounded by a Voronoi cell, which consists of all points that are closer to $x$ than to any other lattice point. The Voronoi cells of $\mathrm{A}_4$ are all identical convex polytopes—can you figure out what this polytope is?
The cells dual to these Voronoi cells are called Delaunay cells. To get the tiling we pick a plane $P$ in 4 dimensions, and whenever $P$ intersects a 2-dimensional face of a Voronoi cell, we project the corresponding 2d face of the corresponding Delaunay cell, which is a triangle, onto $P$. Then we draw these triangles on the plane!
For more details read:
- Peter Kramer, Dual canonical projections.
- Greg Egan, DeBruijn: mathematical details.
Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!
