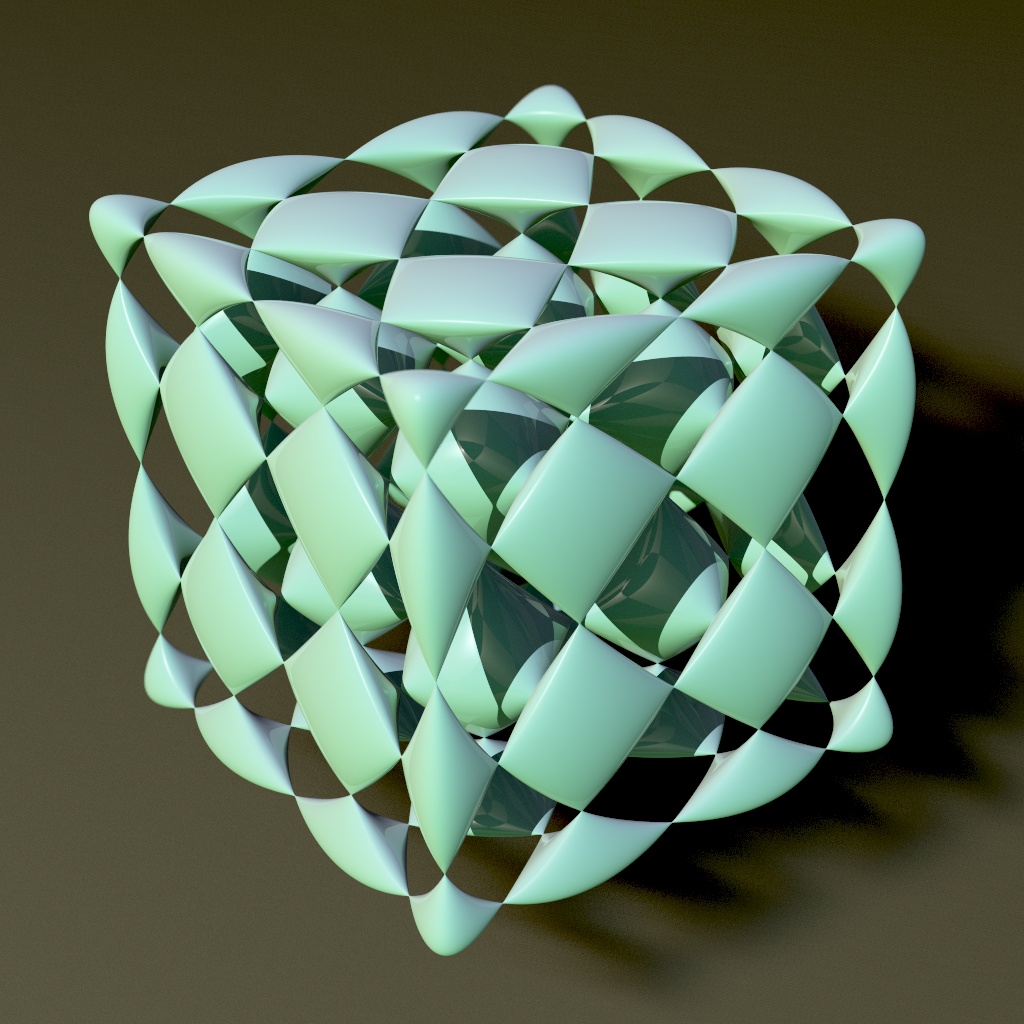
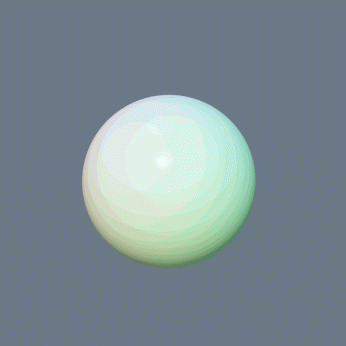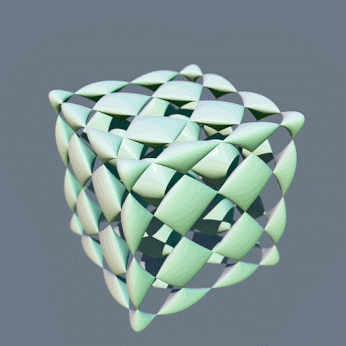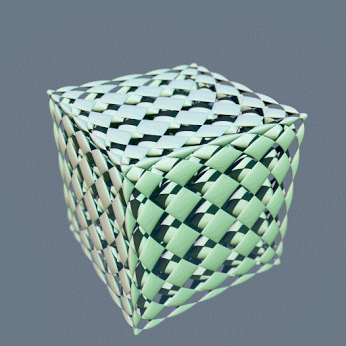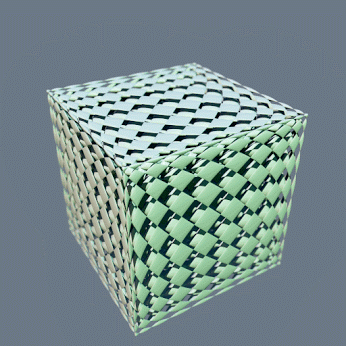
An octic surface is one defined by a polynomial equation of degree 8. This image by Abdelaziz Nait Merzouk shows an octic discovered by Chmutov with 144 real ordinary double points or nodes: that is, points where it looks like the origin of the cone in 3-dimensional space defined by
$$ x^2 + y^2 = z^2 .$$
The Chmutov octic does not have the largest known number of nodes for an octic. That honor currently belongs to the Endrass octic:
which has 168. It is known that an octic with only nodes and no other singularities can have at most 174.
Chmutov defined a series of surfaces with many real nodes with the help of Chebyshev polynomials, in order to establish a lower bound on how many real nodes are possible for a surface of given degree. Here are the Chmutov surfaces of degrees 2 to 20:
and here is the Chmutov icosic, that is, the Chmutov surface of degree 20:
Chmutov also studied surfaces with many complex nodes, including an octic with 154 complex nodes:
• S. V. Chmutov, Examples of projective surfaces with many singularities, J. Algebraic Geom. 1 (1992), 191–196.
but the Chmutov octic with real nodes shown here is discussed in this paper:
• Friedrich Hirzebruch, Some examples of threefolds with trivial canonical bundle. Notes by J. Werner, in Friedrich Hirzebruch, Collected Papers II (1985), pp. 757—770.
where it is used to construct some Calabi—Yau 3-folds.
I thank Juan Escudero for clarifying the difference between the two Chmutov octics. He writes:
The octic with 154 complex nodes belongs to the series discovered by Chmutov in the article “Examples of algebraic surfaces…” (1992). It is a complex surface with equation \(F(u,v,w)=0\) (\(u,v,w \) are complex variables). The real variant of the Chmutov surface with 154 real ordinary double points is obtained with a simple variable change:
$$u=x+iy, v=x-iy, w=z $$
(\(x,y,z\) are real variables) and has equation \(F(x+iy,x-iy,z)=0\), with integer coefficients.
Abdelaziz Nait Merzouk created the above pictures of the Chmutov octic and icosic and made them available on Google+ under a Creative Commons Attribution-ShareAlike 3.0 Unported license. He also put his animated gif of the Chmutov surfaces on Google+ under the same license.
Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!




I’ve made a 3D print : https://www.thingiverse.com/thing:2425953
Cool! I blogged about it on Google+.
> 144 real ordinary double points or nodes: that is, points where it looks like the origin of the cone in 3-dimensional space defined by \(x^2+y^2=z^2\).
The term “double points” seems like a misnomer! That cone looks as if it’s bunching together continuum many points at the origin, not just two.
With real plane curves we say \(y = 0\) intersects \(y = x\) in one point, \(y = x^2 – \epsilon\) intersects \(y = 0\) in two points for \( \epsilon \gt 0\), and \(y = x^2\) intersects \(x = 0\) in a double point: “two points that are infinitesimally far apart”.
With surfaces I guess ‘double point’ is used by analogy.