{7,3,3} Honeycomb Meets Plane at Infinity – Roice Nelson
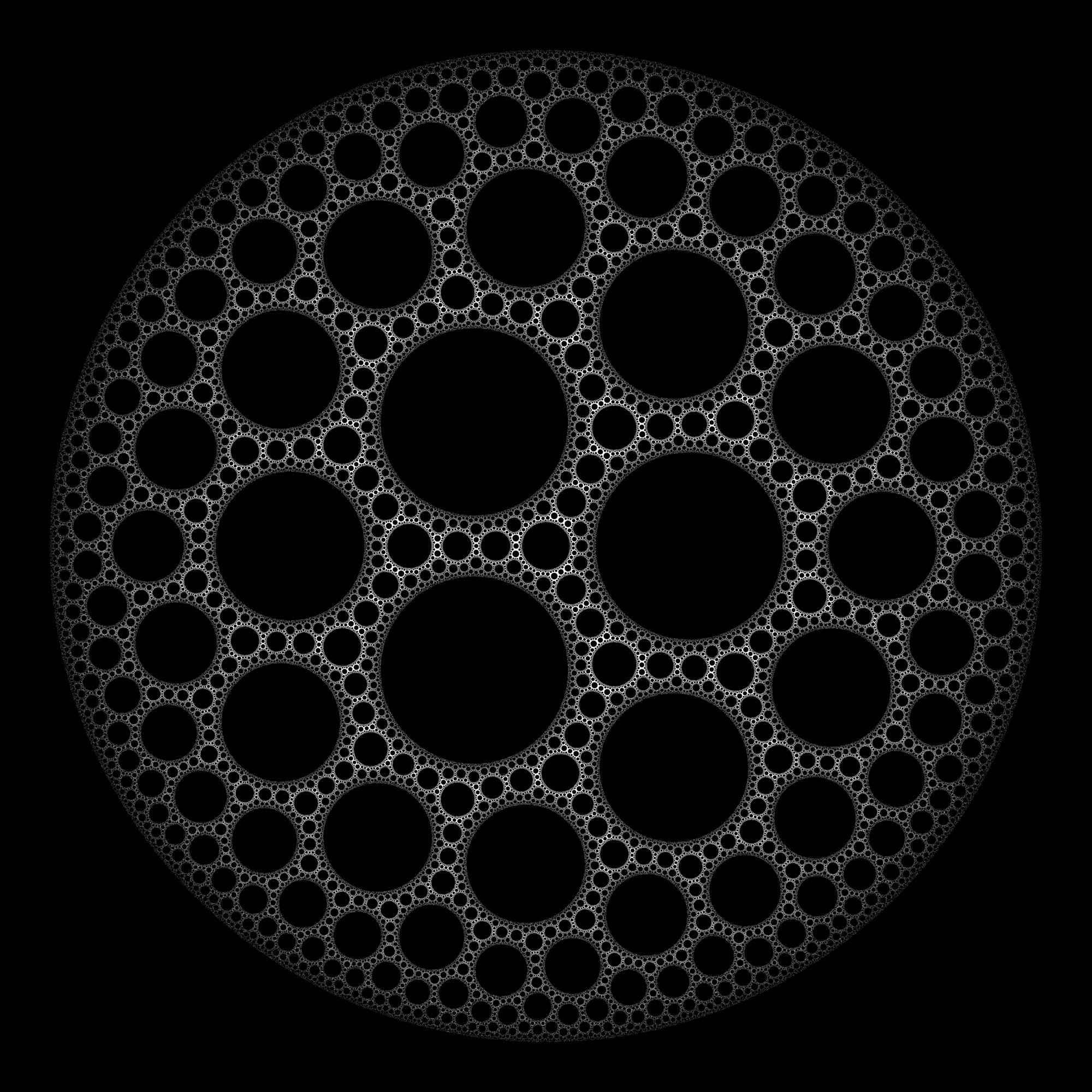
This picture by Roice Nelson shows the boundary of the {7,3,3} honeycomb, the shape featured in our last article:
The black circles are holes, not contained in the boundary of the {7,3,3} honeycomb. There are infinitely many holes, and the actual boundary, shown in white, is a fractal with area zero.
The {7,3,3} honeycomb lives in 3-dimensional hyperbolic space, a space that can be fit inside a ball using the Poincaré ball model. By the ‘boundary’ of the {7,3,3} honeycomb, we mean the set of points on the surface of the Poincaré ball that are limits of points in the {7,3,3} honeycomb.
Roice Nelson used stereographic projection to draw part of the surface of the Poincaré ball as a plane: the plane at infinity. So, the black region on the outside of the picture is also a hole in the boundary of the {7,3,3} honeycomb.
The boundary of the {7,3,3} honeycomb is topologically interesting, because it is homeomorphic to the Sierpinski carpet. See also:
We can see this using a result proved by Gordon Whyburn in 1958. A continuum is a nonempty connected compact metric space. Suppose $X$ is a continuum embedded in the plane. Suppose the complement $\mathbb{R}^2 – X$ has countably many connected components $C_1, C_2, C_3, \dots$ and suppose:
• the diameter of $C_i$ goes to zero as $i \to \infty$;
• the boundary of $C_i$ and the boundary of $C_j$ are disjoint if $i \ne j$;
• the boundary of $C_i$ is a simple closed curve for each $i$;
• the union of the boundaries of $C_i$ is dense in $X$.
Then $X$ is homeomorphic to the Sierpinski carpet!
To apply this result, note that the all the black circles in this picture, and also the black region on the outside, are the connected components $C_i$.
In general, for any $n \ge 7$, the boundary of the {$n$,3,3} honeycomb is homeomorphic to the Sierpinski carpet. For more details, see Section 7 of this paper:
• Danny Calegari and Henry Wilton, 3-manifolds everywhere.
They prove that spaces of this sort occur very often inside the Gromov boundaries of hyperbolic groups.
Roice Nelson, the creator of this image, has a blog with lots of articles about geometry, and he makes plastic models of interesting geometrical objects using a 3d printer:
• Roice.
Visual Insight is a place to share striking images that help explain advanced topics in mathematics. I’m always looking for truly beautiful images, so if you know about one, please drop a comment here and let me know!
